Squaring the Circle Presentation
© Copyright January 1, 2011 By Christopher Ricci; All Rights Reserved
Email Address = [email protected]
Introduction
I never cared much for academics. I graduated from High School by the skin of my teeth. I probably would've failed even at that had it not been for the perseverance of my dear mother. Undoubtedly, of all the subjects I took in school I despised mathematics the most. If someone would've told me back then that I would one day endeavor to solve a problem this complex, I certainly would not have believed them. Frankly, I couldn't care less whether or not the circle could be squared & would not have bothered with it at all had it not been for a single statement I heard about seven years ago. I was talking with an old friend of mine & he brought this subject to my attention. He asserted that even God Himself could not square the circle using only compass & straightedge. The Holy Spirit moved within me the very moment he spoke those words. It was like the Lord was reminding me that "with men this is impossible; but with Him all things are possible." (Matt. 19:26). It was like He had taken that audacious statement as a challenge to His omniscience & was going to prove him wrong; "for with Him nothing [including the squaring of the circle] shall be impossible." (Luke 1:37). Afterall, He created the compass and straightedge & the circle and square. I mean, can this trivial task be any more impossible than splitting the Red Sea or making the sun stand still in the sky? Of course not. There is & can be no doubt about it: God is certainly capable of accomplishing this. And if God can do it then Jesus Christ could do it too. For all power on earth was delivered unto him, (Luke 10:22; John 3:35). And if Christ could do it, then we can do it; with Divine assistance of course. Jesus said that if we had faith as miniscule as a mustard seed we could move mountains, (Matt. 21:21). And through this faith we could do even greater things than he did, (John 14:12). Surely, squaring the circle cannot be any more impossible than walking on water, healing the sick, restoring sight to the blind, or raising the dead! So I embarked on this journey seven years ago in full faith that God would glorify His Holy Name. I prayed fervently along the way for a solution & the following demonstration is the answer I received.
Prelude
It never fails, every time I contemplate the circumference of a circle I am reminded of the mathematical number line. In it we have positive & negative numbers traveling infinitely in opposite directions. They will never meet so long as they travel this way. But don't they also travel infinitely toward each other? The negative numbers can travel endlessly (or decimally) toward the positive, while the positive numbers can travel endlessly (or decimally) toward the negative. No matter how close they get they will never actually collide. Because to do so transforms the negative numbers into the positive & the positive numbers into the negative. And no matter how much the value of zero is crushed between them it will always be there; for it is intuitively obvious that something cannot become nothing. No matter how small something gets it can always be cut in half. The value of zero, therefore, becomes an infinity within itself even though it has circumscribed limitations.
This is precisely why the value of pi has never been ascertained. There is no beginning or ending to the geometrical shape of a circle. For as soon as we assign a beginning to it & draw a perfect arc back upon itself, it can never meet with the point of origin again. As the value of pi infinitely increases the gap between the starting point & the point of destination infinitely decreases. But they will never actually collide. Because to do so transforms the beginning of the arc into the ending & the ending of the arc into the beginning. What we really have then is a ray bent into an arc which has a beginning (only because we've assigned one to it), but no ending, for it can never meet with the point of origin again. For the point of destination to merge with the point of origin is to go too far. The ray overlaps itself; Alpha overtakes Omega; the two become one; either a point is lost or a single point has been counted twice. But for these two points not to merge at all doesn't go far enough & leaves the circle incomplete. This presents a mind-boggling prodigy: It shows that it is possible to have a ray travel INFINITELY toward a point that is directly in its path, and yet NEVER collide with it. It's like the Lord is even able to take eternity, an utterly boundless expanse, place it inside a bottle & simply pop a cork in the top. It retains its infinite, limitless nature despite the fact that it’s confined within a limited finite space. It’s like He sees all of eternity all at once as if encapsulated in a child's snow globe even though there is no beginning or end to it. Friends, I'll tell ya, the Lord never ceases to amaze me!
The frustration man experiences over the value of pi lies within the square. Man can measure the square. But he becomes frustrated because he cannot do the same thing with a circle. He is frustrated for nothing because it stems from the presumption that he could measure the square in the first place. The fact is man cannot measure the perimeter of a square for the very same reason he cannot measure the circumference of a circle. In fact, pi will pose the same problem with all enclosed geometrical figures for they are just circles bent (not broken) into various arcs & angles. Surely, if we take a square swimming pool & fill it with water, the sides will bow out equidistant from the center point & form a perfect circle. Even though this is very easy to visualize & is most certainly true, it is not possible to duplicate the phenomenon geometrically. (So they say). That's because in order to accurately measure any enclosed figure we would need to establish a starting point and the moment we do, we "break" the line & it can never be "reconnected" because pi stands in the way. Inevitably, we run into the very same situation described in the above number line analogy.
To demonstrate my point, try to think of it this way. You have one large square that is divided into 36 squares. There are six rows of six squares. Hence, there are six squares per side of the large square. The perimeter of the large square is 4 sides x 6 squares per side = 24. But if you consider these smaller squares as our unit of measurement, you will find that our calculations for the perimeter are incorrect. You will find that there are only 20 squares around the perimeter; not 24. In multiplying the four sides by the number of squares on each side we've counted the corner squares twice. Every child who has ever tried to build a square box has found this out. The child cuts out a square piece of plywood for the bottom. And then he cuts four boards for the sides; each equal to the side length of the plywood square. But as he desperately struggles to assemble the thing he begins to realize that there is something wrong. The square he has constructed from the four boards is larger than the square piece of plywood. Obviously, this is because he did not take into account the width of the boards. Now this is a very fundamental mistake; but it is perfectly analogous to the situation above. The way we measure squares is fundamentally flawed for in multiplying the measurement of one side by the number of sides, we’ve counted the corner points twice. Surely then, if we begin to build thereupon the finished structure is bound to collapse; for it is founded upon error.
I guess what I'm trying to say is that it is essential that we find a way to thrust the square into the same transcendental world as the circle; for there's no doubt it belongs there. In our quest to accomplish this feat it must be remembered that the square is a "slice" of a cube & is Euclidean in nature; while the circle is a "slice" of a sphere & is spherical in nature. When we take the two of them and lay them side by side within (not upon) a flat surface, we've unwittingly performed a mathematical function. Obviously, the square is perfectly at home there. But we've done injustice to the circle: We've deported it from its comfortable home in spherical geometry, stripped it of its double curvature, & thrust it into flatland; a world utterly foreign to it. If we hope to rectify and/or square the circle in a literal sense we need to balance the equation. We need to give to the square what we took from the circle... and we need to do it "without the square being aware of it." In other words, the square must inherit an intimate relationship with pi, a kind of marriage with the circle... and this must be accomplished without making any visible changes to the diagram. That way it can still be drawn with the primitive instruments at our disposal; namely, a straightedge & unmarked compass. This is essentially what I've done. Consider now, if you will, the following proposal.
This presentation naturally divides itself into Three Parts. They are:
* Part One: Squaring the Circle precisely in Area on Riemannian Manifolds using Plane Geometry.
* Part Two: Circle Rectification precisely in perimeter on Riemannian Manifolds.
* Part Three: Squaring the circle with remarkable accuracy using the Golden Ratio.
Part One
Squaring the Circle precisely on Riemannian Manifolds
Base Diagram
In this first sequence of geometric constructions I will produce a square & a triangle that, when embedded in a smooth Riemannian manifold of positive curvature, will enclose an area precisely equal to pi. I will then produce four additional constructions from each one that are exactly equal to pi... ad infinitum. Afterward, I will demonstrate a technique for producing a rectangle capable of achieving the same result; and will provide four additional shapes derived from it that encompass an area exactly equal to pi. In all, fifteen shapes will be produced to exemplify this remarkable phenomenon.
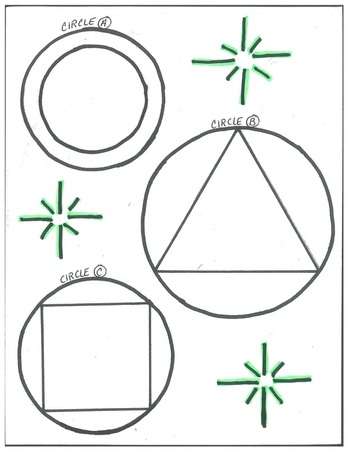
My base diagram consists of three circles on a flat sheet of paper: The top one is labeled Circle A; the middle one is Circle B; and the bottom one is Circle C. Inscribed within these circles are three of the most elementary shapes in all of geometry; a circle, an equilateral triangle, and a square respectively. All six of these figures are related geometrically & can be drawn with compass & straightedge. The technique for their construction will be outlined in forthcoming diagrams. This diagram is all I need to prove that the circle can be squared precisely on Riemannian manifolds using only the primitive tools of plane geometry.
Squaring the Circle precisely on Riemannian Manifolds
Base Diagram
In this first sequence of geometric constructions I will produce a square & a triangle that, when embedded in a smooth Riemannian manifold of positive curvature, will enclose an area precisely equal to pi. I will then produce four additional constructions from each one that are exactly equal to pi... ad infinitum. Afterward, I will demonstrate a technique for producing a rectangle capable of achieving the same result; and will provide four additional shapes derived from it that encompass an area exactly equal to pi. In all, fifteen shapes will be produced to exemplify this remarkable phenomenon.
My base diagram consists of three circles on a flat sheet of paper: The top one is labeled Circle A; the middle one is Circle B; and the bottom one is Circle C. Inscribed within these circles are three of the most elementary shapes in all of geometry; a circle, an equilateral triangle, and a square respectively. All six of these figures are related geometrically & can be drawn with compass & straightedge. The technique for their construction will be outlined in forthcoming diagrams. This diagram is all I need to prove that the circle can be squared precisely on Riemannian manifolds using only the primitive tools of plane geometry.

Circle A
We begin with Circle A, which shall serve as our Unit Circle: Radius = 1; Area = pi.
I will need to inscribe a (Blue) Square within Circle A. It is not part of my base diagram, but it will be used to create several subsequent constructions.
Step #1: Anchor the compass on point [B], and extend it out to point [C], and sweep the Orange Arc counterclockwise as shown.
Step #2: Anchor the compass on point [C], extend it out to point [B], and sweep the Yellow Arc clockwise as illustrated. Label the point of intersection with the letter [X].
Step #3: Draw the Green Line from point [X] down through point [A], and continue the line till it intersects the Red Circle A. Label the two points of intersection with the letters [E] and [D] as shown.
Step #4: Connect points [B-E-C-D] to complete the inscribed Blue Square. According to the Pythagorean Theorem the side lengths of this square would be equal to √2 (1.414213562).
We begin with Circle A, which shall serve as our Unit Circle: Radius = 1; Area = pi.
I will need to inscribe a (Blue) Square within Circle A. It is not part of my base diagram, but it will be used to create several subsequent constructions.
Step #1: Anchor the compass on point [B], and extend it out to point [C], and sweep the Orange Arc counterclockwise as shown.
Step #2: Anchor the compass on point [C], extend it out to point [B], and sweep the Yellow Arc clockwise as illustrated. Label the point of intersection with the letter [X].
Step #3: Draw the Green Line from point [X] down through point [A], and continue the line till it intersects the Red Circle A. Label the two points of intersection with the letters [E] and [D] as shown.
Step #4: Connect points [B-E-C-D] to complete the inscribed Blue Square. According to the Pythagorean Theorem the side lengths of this square would be equal to √2 (1.414213562).
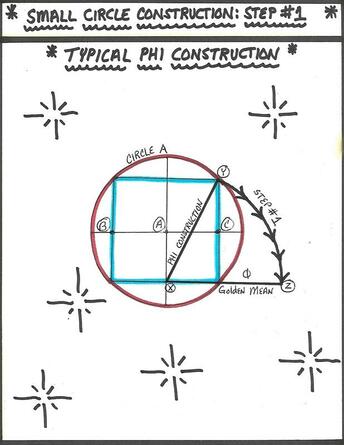
Now I will use that Blue Square to construct the smaller circle inside Circle A. I will erase all unnecessary figures to reduce clutter. I've rotated the diagram 45 degrees to make the construction easier to visualize.
1). Step #1: First, make one typical phi construction using a line segment from the midpoint of the blue square's baseline [X] to the square's vertex [Y]. Label the endpoint with the letter [Z] as shown.
1). Step #1: First, make one typical phi construction using a line segment from the midpoint of the blue square's baseline [X] to the square's vertex [Y]. Label the endpoint with the letter [Z] as shown.
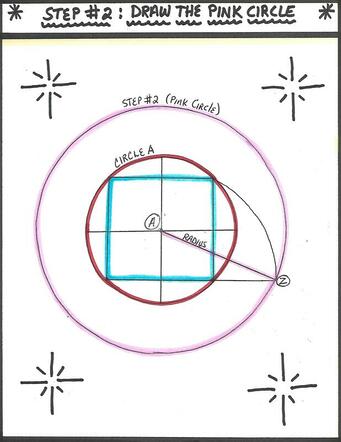
Step #2: Anchor the compass on the center point [A], extend it out to point [Z], and draw the pink circle.
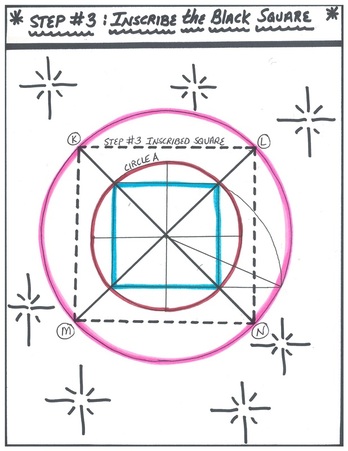
Step #3: Extend the diagonals of the Blue Square outwardly such that they intersect the circumference of the pink circle. Label the points of intersection with the letters [K], [L], [M], and [N] accordingly. Connect these four points to complete an inscribed square.
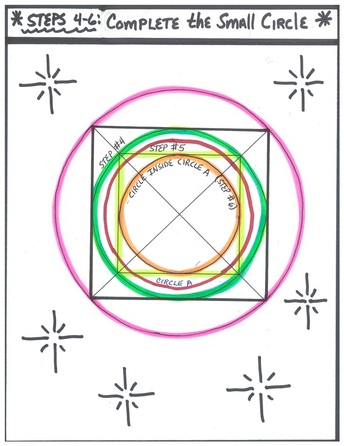
Steps 4-6: Complete the smaller circle within Circle A of my base diagram. I've erased the Blue Square here in order to reduce clutter.
Step #4: Draw the green circle inside the Black Square as described earlier.
Step #5: Inscribe the Yellow Square using the diagonals of the Black Square.
Step #6: Draw the Orange Circle inside the Yellow Square. This completes the construction for the smaller circle inside Circle A depicted in the base diagram. Circle A is exactly 1.3 times the size of this smaller (Orange) Circle.
Step #4: Draw the green circle inside the Black Square as described earlier.
Step #5: Inscribe the Yellow Square using the diagonals of the Black Square.
Step #6: Draw the Orange Circle inside the Yellow Square. This completes the construction for the smaller circle inside Circle A depicted in the base diagram. Circle A is exactly 1.3 times the size of this smaller (Orange) Circle.
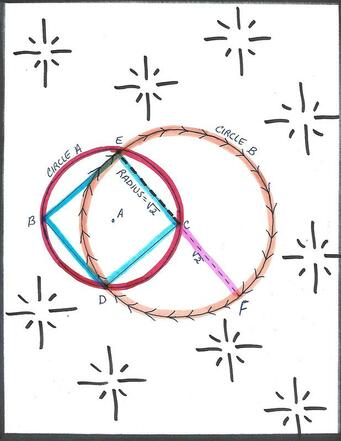
Circle B Construction
We begin with the Red Circle A & the inscribed Blue Square.
Anchor the compass on point [C], extend it out to point [E], and sweep the Orange Arc clockwise for one complete rotation. This is Circle B of my base diagram. It is exactly twice the size of Circle A. Its radius is the square root of two. Finally, extend line segment [EC] to the circle's edge & label the point of intersection with the letter [F] as shown in pink.
We begin with the Red Circle A & the inscribed Blue Square.
Anchor the compass on point [C], extend it out to point [E], and sweep the Orange Arc clockwise for one complete rotation. This is Circle B of my base diagram. It is exactly twice the size of Circle A. Its radius is the square root of two. Finally, extend line segment [EC] to the circle's edge & label the point of intersection with the letter [F] as shown in pink.
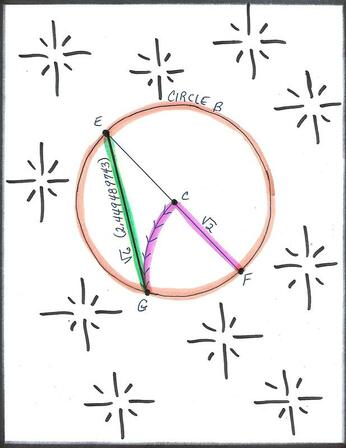
Now to inscribe an equilateral triangle within Circle B as depicted in my base diagram. We begin with Circle B with radius of √2.
Step #1: Anchor the compass on point [F], extend it out to point [C], and sweep the pink arc counterclockwise as shown. Label the point of intersection with the letter [G].
Step #2: Draw the Green Line from point [G] to point [E]. The length of this Green Line would be equal to √6 (2.449489743).
Step #1: Anchor the compass on point [F], extend it out to point [C], and sweep the pink arc counterclockwise as shown. Label the point of intersection with the letter [G].
Step #2: Draw the Green Line from point [G] to point [E]. The length of this Green Line would be equal to √6 (2.449489743).
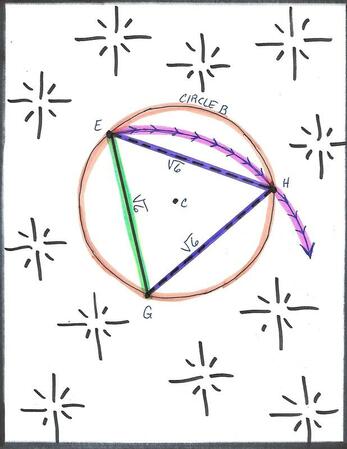
Step #3: Anchor the compass on point [G], extend it out to point [E], and sweep the Pink Arc clockwise until it intersects Circle B. Label that point with the letter [H].
Step #4: Complete the equilateral triangle by connecting points [G-E-H].
Step #4: Complete the equilateral triangle by connecting points [G-E-H].
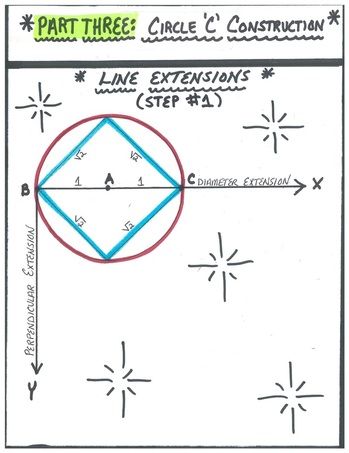
Circle [C] Construction
Again, I will begin with Circle A & the inscribed Blue Square produced earlier.
Step #1: Extend the diameter [BC] outwardly to any random point [X]. Next, extend a right angle line segment down to point [Y] as shown. This is a simple task so I won't bother with its construction.
Again, I will begin with Circle A & the inscribed Blue Square produced earlier.
Step #1: Extend the diameter [BC] outwardly to any random point [X]. Next, extend a right angle line segment down to point [Y] as shown. This is a simple task so I won't bother with its construction.

Step #2 of Circle C construction.
Anchor the compass on point [C], extend it out to point [D], and sweep a green arc counterclockwise such that it intersects the extended diameter line [BX], and label the point of intersection with the letter [E]. Line segment [AE] will have a total length equal to the Silver Ratio (1 + √2 = 2.414213562).
Anchor the compass on point [C], extend it out to point [D], and sweep a green arc counterclockwise such that it intersects the extended diameter line [BX], and label the point of intersection with the letter [E]. Line segment [AE] will have a total length equal to the Silver Ratio (1 + √2 = 2.414213562).
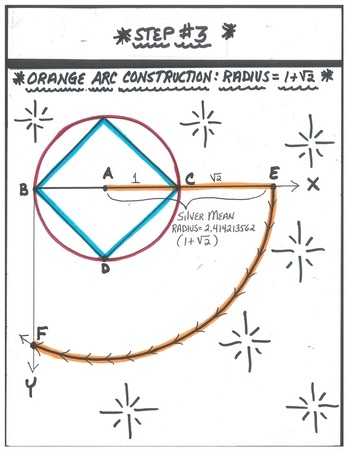
Step #3: Anchor the compass on point [A], extend it out to point [E], and sweep an orange arc clockwise until it intersects with vertical line extension [BY]. Label the point of intersection with the letter [F].

Step #4: The Silver Triangle: The name of this right triangle is obviously derived from the fact that it's hypotenuse is equal in length to the Silver Mean. Here we use the Pythagorean Theorem to calculate the length of segment [BF]. A² - C² = B². The length of line segment [BF] is equal to the square root of that. Hence, the length of this line segment is 2.197368227.
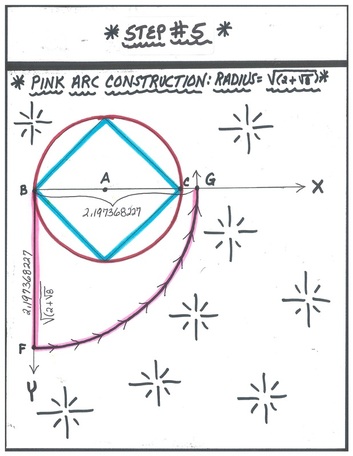
Step #5: Anchor the compass on point [B], extend it out to point [F], and draw the pink arc counterclockwise such that it intersects the extended diameter [BX]. Label the point of intersection with the letter [G] accordingly. Line segment [BG] and line segment [BF] are now both equal in length, (2.197368227).
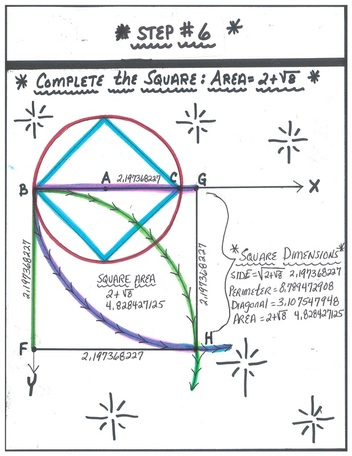
Step #6: Now I will complete the square within which Circle C shall be drawn. I have determined two sides of this square in previous steps. The remaining two sides are constructed as follows.
1). Anchor the compass on point [F], extend it out to point [B], and sweep a green arc clockwise.
2). Anchor the compass on point [G], extend it out to point [B], and sweep a purple arc counterclockwise such that the two arcs intersect at point [H].
3). Connect points [GH] & points [FH] with line segments as depicted. Now we have a completed square with side lengths of 2.197368227; which is the square root of 2X the Silver Mean.
1). Anchor the compass on point [F], extend it out to point [B], and sweep a green arc clockwise.
2). Anchor the compass on point [G], extend it out to point [B], and sweep a purple arc counterclockwise such that the two arcs intersect at point [H].
3). Connect points [GH] & points [FH] with line segments as depicted. Now we have a completed square with side lengths of 2.197368227; which is the square root of 2X the Silver Mean.

Step #7: Now to complete Circle C of the base diagram. Join the vertices of the square constructed in the previous frame with St. Andrew's Cross to determine the center point; label it with the letter [I]. Anchor the compass there, extend it out to the midpoint of the square, and draw the Orange Circle. This completes the construction of Circle C.
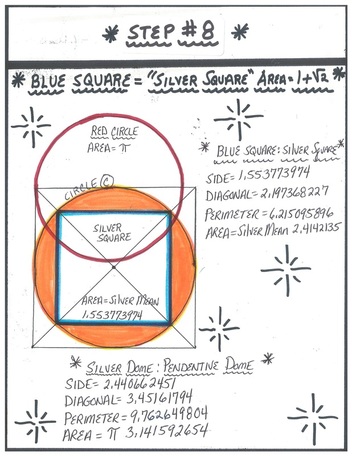
Step #8: Complete the inscribed square within Circle C as illustrated in the base diagram. Here, we simply repeat the procedure outlined in previous frames. Join the four points where the diagonals of the larger square intersect the circumference of the orange Circle C with line segments. The inscribed square is now complete. I often refer to this as the "Silver Square" since its area in flatland is equal to the Silver Mean.
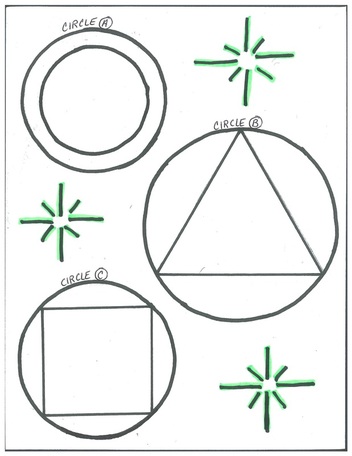
Now, I have constructed all six figures represented in the base diagram. Considering Circle A as a unit circle, the following calculations will result.
* Circle A has a diameter length of 2; Radius = 1; Area = pi. Circle A is exactly 1.3 times the size of the smaller circle inside. The smaller inscribed circle has a circumference of 5.441398093. As far as this smaller circle is concerned, this is the only measurement of any importance to us here because it is unaffected by the manifold; it remains constant whether it is embedded within a Euclidean or spherical surface.
* Circle B is twice the size of Circle A. It has a diameter length of √8 = (2.828427125); Radius = √2 (1.414213562); It's Area = 2 pi.
* Circle C has a diameter length of the square root of those two diameters added together: The sq.rt. of (2 + √8) = 2.197368227; Radius = 1.0986841; It’s area = 1/2pi x Silver Mean = 3.792237796.
* Circle A has a diameter length of 2; Radius = 1; Area = pi. Circle A is exactly 1.3 times the size of the smaller circle inside. The smaller inscribed circle has a circumference of 5.441398093. As far as this smaller circle is concerned, this is the only measurement of any importance to us here because it is unaffected by the manifold; it remains constant whether it is embedded within a Euclidean or spherical surface.
* Circle B is twice the size of Circle A. It has a diameter length of √8 = (2.828427125); Radius = √2 (1.414213562); It's Area = 2 pi.
* Circle C has a diameter length of the square root of those two diameters added together: The sq.rt. of (2 + √8) = 2.197368227; Radius = 1.0986841; It’s area = 1/2pi x Silver Mean = 3.792237796.

Change in Manifold
Now, I change the Euclidean surface to a smooth Riemannian manifold of positive Ricci curvature & exemplify this by darkening the background with a black magic marker. Please note: The base diagram itself has not changed; in fact this is a photocopy. All six figures remain the same & can still be drawn with the primitive instruments of Plane Geometry; namely, an unmarked compass & straightedge.
To demonstrate what I'm talking about imagine you walk outside in the middle of the night & gaze up into the pitch black sky and notice three full moons (i.e., hemispheres); each with its own unique shape inscribed within its equator circumference: In other words, the image you see in the night sky is exactly like the one appearing here on your screen.
Taking into consideration the aforementioned calculations, the circle is squared with exact precision. Under these conditions both the inscribed equilateral triangle and the inscribed square encompass a total amount of surface area EXACTLY equal to pi:
(3.14159265358979323846264338327950288419716939937510582097494459...).
Now, this value is not just "close" to that of pi; like to the trillionth decimal point... or to the trillion trillionth... or to any other finite number... but is accurate TO INFINITY. This is a profound discovery; for the triangle & the square both now share the transcendental nature of the circle. They both enclose the same amount of curved surface area as that which is bound by the smaller inscribed circle on Hemisphere [A]. They also enclose the same amount of flat surface area encompassed by Circle A; with both being pi exactly.
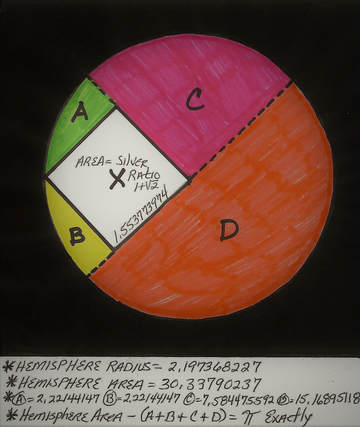
Several other neat things can be observed here. Since we are dealing with hemispheres rather than flat circles, their respective areas have now doubled in size. The area of Hemisphere [A] is now 2pi; the inscribed circle dividing the hemisphere’s surface into two separate sectors exactly equal to pi each. The area of hemisphere [B] is now 4pi; the inscribed triangle dividing its surface into four separate sectors with each one being exactly equal to pi. Hemisphere [C] is especially intriguing: The area has now doubled in size. Its surface area is equal to pi x the Silver Ratio (7.584475592), and the area bound by the square is pi exactly. The interesting thing about this particular diagram is that each of the four sectors lying outside the perimeter of the square encompass an area exactly equal to the Form Factor Ratio. (See the diagrams below for a breakdown of their respective dimensions).
Perhaps this would be easier to visualize if we think of it another way: Imagine you have three hemispherical lumps of cookie dough sitting flat-side down on a table; each one identical in size to those depicted here on your screen. Now, imagine you have three differently shaped cookie cutters (one circular, another triangular, and the other square); each identical in size to those illustrated here. You then take each cutter & center it directly above its corresponding hemisphere & press each one down into the surface such that they sink all the way down to the table, level with the equator circle. You will find that the vertices of the triangular cutter will come into contact with the equator circle at three equidistant points on the table; slicing off three half-caps from the hemisphere; each with the same dimensions as those stated above. The vertices of the square, too, will come into contact with the hemisphere's equator circle at four equidistant points; severing four half-caps from the lump of dough; each one identical in size & shape to those delineated above. After you subtract the amount of surface area of those half-caps from that of their respective hemispheres, you will find that the amount of area left over on the surface of each one of them is exactly equal to pi.
Now, I change the Euclidean surface to a smooth Riemannian manifold of positive Ricci curvature & exemplify this by darkening the background with a black magic marker. Please note: The base diagram itself has not changed; in fact this is a photocopy. All six figures remain the same & can still be drawn with the primitive instruments of Plane Geometry; namely, an unmarked compass & straightedge.
To demonstrate what I'm talking about imagine you walk outside in the middle of the night & gaze up into the pitch black sky and notice three full moons (i.e., hemispheres); each with its own unique shape inscribed within its equator circumference: In other words, the image you see in the night sky is exactly like the one appearing here on your screen.
Taking into consideration the aforementioned calculations, the circle is squared with exact precision. Under these conditions both the inscribed equilateral triangle and the inscribed square encompass a total amount of surface area EXACTLY equal to pi:
(3.14159265358979323846264338327950288419716939937510582097494459...).
Now, this value is not just "close" to that of pi; like to the trillionth decimal point... or to the trillion trillionth... or to any other finite number... but is accurate TO INFINITY. This is a profound discovery; for the triangle & the square both now share the transcendental nature of the circle. They both enclose the same amount of curved surface area as that which is bound by the smaller inscribed circle on Hemisphere [A]. They also enclose the same amount of flat surface area encompassed by Circle A; with both being pi exactly.
Several other neat things can be observed here. Since we are dealing with hemispheres rather than flat circles, their respective areas have now doubled in size. The area of Hemisphere [A] is now 2pi; the inscribed circle dividing the hemisphere’s surface into two separate sectors exactly equal to pi each. The area of hemisphere [B] is now 4pi; the inscribed triangle dividing its surface into four separate sectors with each one being exactly equal to pi. Hemisphere [C] is especially intriguing: The area has now doubled in size. Its surface area is equal to pi x the Silver Ratio (7.584475592), and the area bound by the square is pi exactly. The interesting thing about this particular diagram is that each of the four sectors lying outside the perimeter of the square encompass an area exactly equal to the Form Factor Ratio. (See the diagrams below for a breakdown of their respective dimensions).
Perhaps this would be easier to visualize if we think of it another way: Imagine you have three hemispherical lumps of cookie dough sitting flat-side down on a table; each one identical in size to those depicted here on your screen. Now, imagine you have three differently shaped cookie cutters (one circular, another triangular, and the other square); each identical in size to those illustrated here. You then take each cutter & center it directly above its corresponding hemisphere & press each one down into the surface such that they sink all the way down to the table, level with the equator circle. You will find that the vertices of the triangular cutter will come into contact with the equator circle at three equidistant points on the table; slicing off three half-caps from the hemisphere; each with the same dimensions as those stated above. The vertices of the square, too, will come into contact with the hemisphere's equator circle at four equidistant points; severing four half-caps from the lump of dough; each one identical in size & shape to those delineated above. After you subtract the amount of surface area of those half-caps from that of their respective hemispheres, you will find that the amount of area left over on the surface of each one of them is exactly equal to pi.

Hemisphere B: Total surface area = 4pi (12.56637061). The height of each of the three spherical caps (A, B, & C) lying outside the perimeter of the triangle = .707106781. This means the surface area of each cap is 6.283185307; which is 2pi. Divided in half for the hemisphere = pi exactly. Therefore, the total hemisphere area (4pi) minus the area of the three half-caps lying outside the triangle (3pi) = the surface area bound by the triangle {X}, which is pi exactly.
Since we now know that an equilateral triangle will divide the hemisphere's curved surface area into four equal sectors, other polygons can be constructed from this diagram that will also be equal to pi. See the diagrams below for examples of this extraordinary phenomenon.
Since we now know that an equilateral triangle will divide the hemisphere's curved surface area into four equal sectors, other polygons can be constructed from this diagram that will also be equal to pi. See the diagrams below for examples of this extraordinary phenomenon.
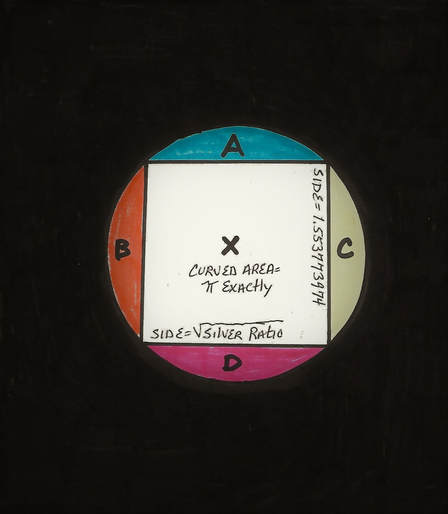
Hemisphere C: Total hemispherical surface area is equal to pi x the Silver Ratio (7.584475592). The height of each of the four separate spherical caps (A, B, C, & D) lying outside the perimeter of the square is .321797127. Hence, the surface area bound by each cap is equal to 2.221441469. Dividing that in half for the hemisphere = The Form Factor Ratio (1.110720735). The sum of these four half-caps = 4.442882938; which is pi times √2 ... subtracting that figure from the total amount of hemispherical surface area (7.584475592) = the area of the square {X}, which is pi exactly.
Incidentally, this Form Factor Ratio is an integral part of our everyday lives: It is used in electronics like television, radio, computers, cell phones, etc to convert sinusoidal (i.e., curved) waves into digital (i.e., square) waves; in essence, squaring the circle. It is no coincidence that this square is a perfect blueprint for the sail vault & pendentive dome atop the Byzantine Church, Hagia Sophia. Please examine the diagram below to see what this would look like from a side view.
Since we now know the area of this square (i.e., Sail Vault) is equal to pi exactly, the following observations become apparent:
Sail Vault Area (pi)/√2 = Area of each of the four caps lying outside the square (2.221441469).
Hemisphere Area/Silver Ratio = Area of the Sail Vault = (pi exactly).
Hemisphere Area/[1 + the Silver Ratio] (3.414213562) = Area of each of the four caps lying outside the square (2.221441469).
Incidentally, this Form Factor Ratio is an integral part of our everyday lives: It is used in electronics like television, radio, computers, cell phones, etc to convert sinusoidal (i.e., curved) waves into digital (i.e., square) waves; in essence, squaring the circle. It is no coincidence that this square is a perfect blueprint for the sail vault & pendentive dome atop the Byzantine Church, Hagia Sophia. Please examine the diagram below to see what this would look like from a side view.
Since we now know the area of this square (i.e., Sail Vault) is equal to pi exactly, the following observations become apparent:
Sail Vault Area (pi)/√2 = Area of each of the four caps lying outside the square (2.221441469).
Hemisphere Area/Silver Ratio = Area of the Sail Vault = (pi exactly).
Hemisphere Area/[1 + the Silver Ratio] (3.414213562) = Area of each of the four caps lying outside the square (2.221441469).

This is what the "square" on Hemisphere {C} would look like from the side. From directly above it looks like a perfectly flat square centered within a perfectly flat circle, but from the side it resembles a square sheet draped over a hemisphere. Each of the four corners come into contact with the hemisphere's equator circle at four equidistant points. Looking straight down on it from directly above, (or straight up at it from directly below, as would be the case if this were engraved upon the surface of the moon), every single point on its surface is visible, but curvature is completely undetectable. The equilateral triangle upon Hemisphere {B} would have a similar appearance; except that its vertices would come into contact at three equidistant points on the equator circle, rather than four. This is but a brief glimpse at the sail vault & its relationship to the subject at hand. For additional diagrams & the dimensions of this object please see Part Two of this demonstration.
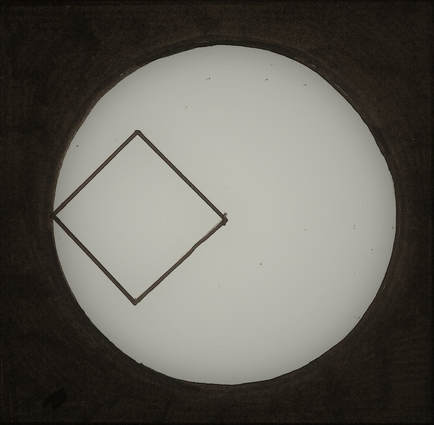
Other Polygons derived from the Square within Circle [C]
Since it has been proven that the surface area encompassed by the square in Circle [C] is pi exactly, other polygons that encompass an area of pi exactly can be derived therefrom by dividing that square in half, or in fourths. Naturally, the size of the hemispheres upon which these polygons appear will have to be increased accordingly. If we cut the square in half horizontally or diagonally, the size of the hemisphere has to be doubled. If we cut it into fourths, the size of the hemisphere must increase by four. Since we already have the base diagram to work from, accomplishing this is so simple that I am not going to bother providing the techniques for their construction. Here are some examples.
Please Note: When the diagram falls entirely on one side of a Great Circle, certain qualities are lost. For example, opposite sides are no longer equal in length.
Since it has been proven that the surface area encompassed by the square in Circle [C] is pi exactly, other polygons that encompass an area of pi exactly can be derived therefrom by dividing that square in half, or in fourths. Naturally, the size of the hemispheres upon which these polygons appear will have to be increased accordingly. If we cut the square in half horizontally or diagonally, the size of the hemisphere has to be doubled. If we cut it into fourths, the size of the hemisphere must increase by four. Since we already have the base diagram to work from, accomplishing this is so simple that I am not going to bother providing the techniques for their construction. Here are some examples.
Please Note: When the diagram falls entirely on one side of a Great Circle, certain qualities are lost. For example, opposite sides are no longer equal in length.
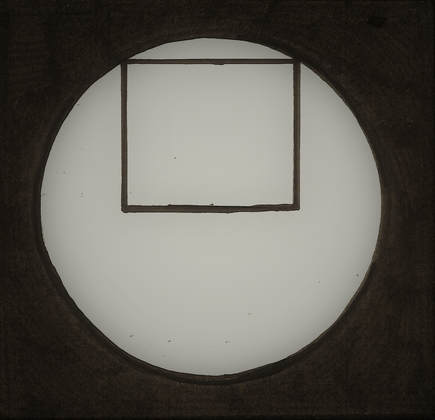
Here, I've cut the square in half horizontally; thereby producing a rectangle... and doubled the size of the hemisphere.
Imagine you walk outside on a dark night & look up at a full moon; which is actually one-half of the moon; a hemisphere. By definition this is a two-dimensional surface & from blueprint perspective you can see every single point on its surface. From earth it looks like a flat white circle. But upon this particular night you notice a rectangle etched into its surface…. In other words, the vision you see in the night sky is exactly like the one appearing here on your screen.
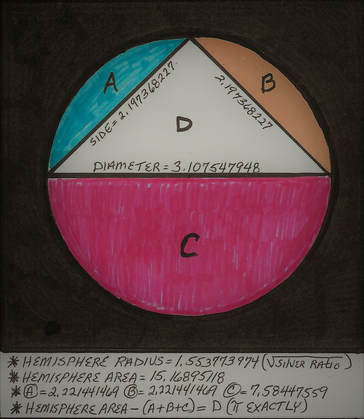
If the full moon was a perfect hemisphere & its radius was the square root of the Silver Ratio (1.553773974), then the area encompassed by the rectangle would be exactly equal to pi… ad infinitum. The chord height for all three sectors {A, B & C} is the same; .455089861. Therefore, their combined area is equal to 4.442882942; which is four times the Form Factor Ratio. The chord height of area {D} is equal to the radius length, which means that its surface area is precisely ½ the total area of the hemisphere; 7.584475572. The sum of all four sectors (A + B + C + D) lying outside the rectangle is 12.02735853. Subtracting that figure from the hemisphere’s total surface area will give you the exact amount of surface area {X} encompassed by the rectangle. 15.16895118 – 12.02735853 = 3.141592654; which is pi exactly.
Imagine you walk outside on a dark night & look up at a full moon; which is actually one-half of the moon; a hemisphere. By definition this is a two-dimensional surface & from blueprint perspective you can see every single point on its surface. From earth it looks like a flat white circle. But upon this particular night you notice a rectangle etched into its surface…. In other words, the vision you see in the night sky is exactly like the one appearing here on your screen.
If the full moon was a perfect hemisphere & its radius was the square root of the Silver Ratio (1.553773974), then the area encompassed by the rectangle would be exactly equal to pi… ad infinitum. The chord height for all three sectors {A, B & C} is the same; .455089861. Therefore, their combined area is equal to 4.442882942; which is four times the Form Factor Ratio. The chord height of area {D} is equal to the radius length, which means that its surface area is precisely ½ the total area of the hemisphere; 7.584475572. The sum of all four sectors (A + B + C + D) lying outside the rectangle is 12.02735853. Subtracting that figure from the hemisphere’s total surface area will give you the exact amount of surface area {X} encompassed by the rectangle. 15.16895118 – 12.02735853 = 3.141592654; which is pi exactly.
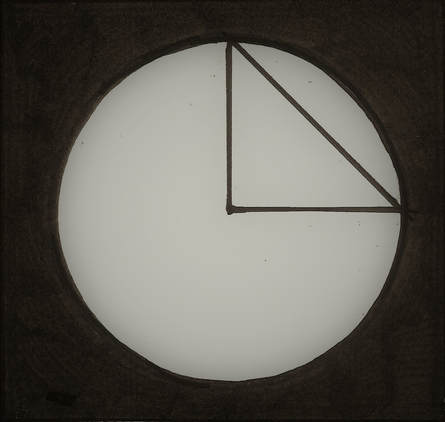
Here, I've divided the square in half diagonally; thereby producing an isosceles right triangle... and doubled the hemisphere's size.
If the full moon was a perfect hemisphere & its radius was the square root of the Silver Ratio (1.553773974), then the area encompassed by the triangle would be exactly equal to pi… ad infinitum. The chord height of each of the half-caps {A & B} is .455089861. Therefore, the area of each one is twice the Form Factor Ratio; 2.221441471. The two added together is equal to 4.442882942. The chord height of area {C} is equal to the radius length, which means that its surface area is precisely ½ the total area of the hemisphere; 7.584475572. The sum of all three half-caps (A + B + C) lying outside the triangle is 12.02735853. Subtracting that figure from the hemisphere’s total surface area will give you the exact amount of surface area {D} encompassed within the triangle. 15.16895118 – 12.02735853 = 3.141592654; which is pi exactly.
If the full moon was a perfect hemisphere & its radius was the square root of the Silver Ratio (1.553773974), then the area encompassed by the triangle would be exactly equal to pi… ad infinitum. The chord height of each of the half-caps {A & B} is .455089861. Therefore, the area of each one is twice the Form Factor Ratio; 2.221441471. The two added together is equal to 4.442882942. The chord height of area {C} is equal to the radius length, which means that its surface area is precisely ½ the total area of the hemisphere; 7.584475572. The sum of all three half-caps (A + B + C) lying outside the triangle is 12.02735853. Subtracting that figure from the hemisphere’s total surface area will give you the exact amount of surface area {D} encompassed within the triangle. 15.16895118 – 12.02735853 = 3.141592654; which is pi exactly.
Here, I've divided the square into fourths & increased the size of the hemisphere by four.
If the full moon was a perfect hemisphere & its radius was 2.197368227; and the area of the square was equal to the Silver Ratio; and it was situated precisely as shown on this diagram, then the total amount of the hemisphere’s surface area encompassed thereby would be exactly equal to pi. The chord height of Area {A} is .643594253; its area is twice the Form Factor Ratio = 2.22144147. The chord height & area of sector {B} is identical to that of sector {A}; its area is also 2.22144147. The chord height of sector {C} is the radius; its area is equal to ¼ the hemisphere’s total surface area = 7.584475592. The chord height of sector {D} is also the radius length; its area is equal to ½ the hemisphere’s total area = 15.16895118. The total amount of hemispherical area lying outside the square (A + B + C + D) = 27.19630972. Subtract that from the hemisphere’s total area = the area bound by the square {X}; which is pi exactly.
If the full moon was a perfect hemisphere & its radius was 2.197368227; and the area of the square was equal to the Silver Ratio; and it was situated precisely as shown on this diagram, then the total amount of the hemisphere’s surface area encompassed thereby would be exactly equal to pi. The chord height of Area {A} is .643594253; its area is twice the Form Factor Ratio = 2.22144147. The chord height & area of sector {B} is identical to that of sector {A}; its area is also 2.22144147. The chord height of sector {C} is the radius; its area is equal to ¼ the hemisphere’s total surface area = 7.584475592. The chord height of sector {D} is also the radius length; its area is equal to ½ the hemisphere’s total area = 15.16895118. The total amount of hemispherical area lying outside the square (A + B + C + D) = 27.19630972. Subtract that from the hemisphere’s total area = the area bound by the square {X}; which is pi exactly.
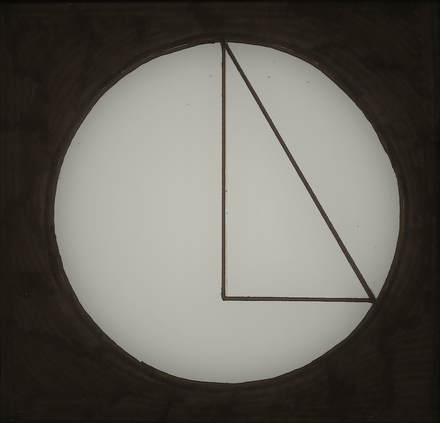
Here again, I've cut the square into fourths & increased the hemisphere's size by four.
If the full moon was a perfect hemisphere & its radius was the square root of 2X the Silver Ratio (2.197368227); and the dimensions of the triangle were identical to those delineated here; and it was situated precisely as shown on this diagram, then the total amount of the hemisphere’s surface area encompassed thereby would be exactly equal to pi. The chord height of Area {A} is the radius; it encompasses ¼ the hemisphere’s total area = 7.584475592. The chord height of sector {B} is .643594253. Hence, the total amount of area encompassed thereby is 4X the Form Factor Ratio = 4.44288294. The chord height of sector {C} is the radius; its area is exactly ½ the hemisphere’s total area = 15.16895118. The total amount of the hemisphere’s surface area lying outside the triangle (A + B + C) = 27.19630972. Subtracting that sum from the hemisphere’s total area will provide the amount of area encompassed within triangle {X}; which is pi exactly.
Any line drawn through the center of the circle (or hemisphere) will cut the square in half such that both halves will be identical in size & shape, and both halves will be situated in precisely the same position. Since there is an unlimited number of ways to do this, there are an unlimited number of shapes that can be constructed therefrom with each half being exactly equal to pi (that is, so long as the size of the hemisphere is doubled in area).
Some will argue that what I've drawn is not actually a square. Some may cry “foul.” Others may call it a trick, or an illusion. Some will assert that I bent the rules. But that is not so. I did not bend the rules, I bent the lines, or more specifically, the surface upon which they appear; albeit, the curvature is impossible to detect from this angle. As Einstein once said: "If the facts don't fit the theory, change the facts." That's kinda what I did, except I didn't actually "change" them; I merely looked at them from a different perspective. Hence, these diagrams are constructible via compass & straightedge. Both squares (whether curved or flat) share nearly all the same characteristics. They are both embedded in a two-dimensional manifold & every single point on the surface (whether curved or flat) is visible. For example: If you look straight down on a hemisphere you can see every single point on its surface (much like a full moon); it's just that, although the points are evenly distributed, they appear to be crushed closer & closer together as they near the equator circle. The same thing happens when viewing a flat plane from a 45 degree angle... every point on its surface is still visible & evenly distributed; except that the points on its surface that are closeest to you will appear evenly distributed, while those farthest from you will seem to be crushed closer & closer together the farther away they are from your viewpoint. All four sides of both squares (curved or flat) are equal in length. The perimeter is equal to four times the length of one side. The four sides intersect at right angles on the equator circle. The diagonals (whether curved or flat) are equal in length; they bisect each other; they meet at 90° angles; they bisect the angles of the four corners; and they still obey the Pythagorean Theorem. And, for all intents & purposes, opposite sides are "parallel." In other words, if you draw lines of longitude (i.e., great circles) through both sides of the "square," they will intersect its sides orthogonally much like the lines of latitude on opposite sides of the equator circle, and all points on one side of the square remain equidistant from their corresponding points on the opposite side throughout their respective lengths.
If the full moon was a perfect hemisphere & its radius was the square root of 2X the Silver Ratio (2.197368227); and the dimensions of the triangle were identical to those delineated here; and it was situated precisely as shown on this diagram, then the total amount of the hemisphere’s surface area encompassed thereby would be exactly equal to pi. The chord height of Area {A} is the radius; it encompasses ¼ the hemisphere’s total area = 7.584475592. The chord height of sector {B} is .643594253. Hence, the total amount of area encompassed thereby is 4X the Form Factor Ratio = 4.44288294. The chord height of sector {C} is the radius; its area is exactly ½ the hemisphere’s total area = 15.16895118. The total amount of the hemisphere’s surface area lying outside the triangle (A + B + C) = 27.19630972. Subtracting that sum from the hemisphere’s total area will provide the amount of area encompassed within triangle {X}; which is pi exactly.
Any line drawn through the center of the circle (or hemisphere) will cut the square in half such that both halves will be identical in size & shape, and both halves will be situated in precisely the same position. Since there is an unlimited number of ways to do this, there are an unlimited number of shapes that can be constructed therefrom with each half being exactly equal to pi (that is, so long as the size of the hemisphere is doubled in area).
Some will argue that what I've drawn is not actually a square. Some may cry “foul.” Others may call it a trick, or an illusion. Some will assert that I bent the rules. But that is not so. I did not bend the rules, I bent the lines, or more specifically, the surface upon which they appear; albeit, the curvature is impossible to detect from this angle. As Einstein once said: "If the facts don't fit the theory, change the facts." That's kinda what I did, except I didn't actually "change" them; I merely looked at them from a different perspective. Hence, these diagrams are constructible via compass & straightedge. Both squares (whether curved or flat) share nearly all the same characteristics. They are both embedded in a two-dimensional manifold & every single point on the surface (whether curved or flat) is visible. For example: If you look straight down on a hemisphere you can see every single point on its surface (much like a full moon); it's just that, although the points are evenly distributed, they appear to be crushed closer & closer together as they near the equator circle. The same thing happens when viewing a flat plane from a 45 degree angle... every point on its surface is still visible & evenly distributed; except that the points on its surface that are closeest to you will appear evenly distributed, while those farthest from you will seem to be crushed closer & closer together the farther away they are from your viewpoint. All four sides of both squares (curved or flat) are equal in length. The perimeter is equal to four times the length of one side. The four sides intersect at right angles on the equator circle. The diagonals (whether curved or flat) are equal in length; they bisect each other; they meet at 90° angles; they bisect the angles of the four corners; and they still obey the Pythagorean Theorem. And, for all intents & purposes, opposite sides are "parallel." In other words, if you draw lines of longitude (i.e., great circles) through both sides of the "square," they will intersect its sides orthogonally much like the lines of latitude on opposite sides of the equator circle, and all points on one side of the square remain equidistant from their corresponding points on the opposite side throughout their respective lengths.
Other polygons derived from the Triangle within Circle [B]
Now, I intend to do the same thing with the Triangle within Circle [B] of my initial presentation. Again, the size of each hemisphere will have to be adjusted accordingly. If I cut the triangle in half I will double the hemisphere's size. If I cut it into thirds I will triple it; and if into sixths I will increase the hemisphere's area by six. This is actually easier to achieve & prove since the triangle divides the hemisphere into four sectors with the same amount of surface area. Remarkably, I have never heard this before, nor read it in any book.
Now, I intend to do the same thing with the Triangle within Circle [B] of my initial presentation. Again, the size of each hemisphere will have to be adjusted accordingly. If I cut the triangle in half I will double the hemisphere's size. If I cut it into thirds I will triple it; and if into sixths I will increase the hemisphere's area by six. This is actually easier to achieve & prove since the triangle divides the hemisphere into four sectors with the same amount of surface area. Remarkably, I have never heard this before, nor read it in any book.
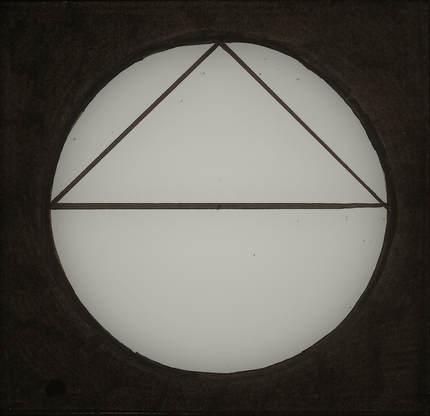
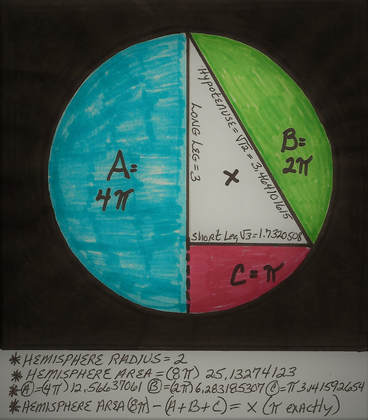
Here, I have divided the Equilateral Triangle in half & doubled the size of the hemisphere; thereby producing a large 1-2-3 Right Triangle.
Imagine you walk outside on a dark night & look up at a full moon; which is actually one-half of the moon; a hemisphere. By definition this is a two-dimensional surface & from blueprint perspective you can see every single point on its surface. From earth it looks like a flat white circle. But upon this particular night you notice a 1-2-3 Right triangle etched into its surface…. In other words, the vision you see in the night sky is exactly like the one appearing here on your screen.
If the moon was a perfect sphere & its radius was 2, then the area encompassed by the triangle would be exactly equal to pi. The chord height of Area {A} is 2; its surface area is 4pi (12.56637061). Chord height of Area {B} is 1; its surface area is 2pi (6.283185307). The chord height of Area {C} is also 1; but its area is ½ that of Area {B}. So its area is 1pi (3.141592654). The sum of these three areas (A + B + C) = 21.99114858 (7pi). The area of the entire hemisphere is 8pi. Hemisphere area (25.13274123) – the area lying outside the triangle (21.99114858) = the area enclosed by the triangle {X} which is pi exactly; (3.141592654).
Imagine you walk outside on a dark night & look up at a full moon; which is actually one-half of the moon; a hemisphere. By definition this is a two-dimensional surface & from blueprint perspective you can see every single point on its surface. From earth it looks like a flat white circle. But upon this particular night you notice a 1-2-3 Right triangle etched into its surface…. In other words, the vision you see in the night sky is exactly like the one appearing here on your screen.
If the moon was a perfect sphere & its radius was 2, then the area encompassed by the triangle would be exactly equal to pi. The chord height of Area {A} is 2; its surface area is 4pi (12.56637061). Chord height of Area {B} is 1; its surface area is 2pi (6.283185307). The chord height of Area {C} is also 1; but its area is ½ that of Area {B}. So its area is 1pi (3.141592654). The sum of these three areas (A + B + C) = 21.99114858 (7pi). The area of the entire hemisphere is 8pi. Hemisphere area (25.13274123) – the area lying outside the triangle (21.99114858) = the area enclosed by the triangle {X} which is pi exactly; (3.141592654).

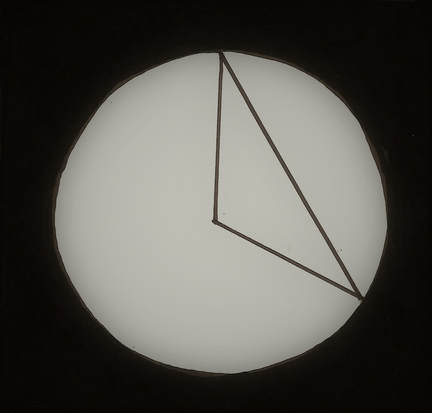
I've decided the best way to proceed from here is to divide both the Triangle & the hemisphere into six equal sectors. This is easily done by drawing a line from each vertex straight through the opposite side of the Triangle to the equator circle. The Orange Isosceles Triangle occupies two of these sectors (or 1/3). The kite-shaped Pink Tetragon also occupies two sectors (or 1/3). And the little Yellow Right Triangle is confined within only one sector (or 1/6). This will allow me to quickly & easily demonstrate & prove my work.
Here, I have isolated the Orange Isosceles Triangle & increased the hemisphere's size by three.
Since the hemisphere is 12pi & is divided into thirds, the area of Sector {A} is 12.56637061 (4pi). The area of Sector {B} is also one third of the hemisphere's total surface area = 12.56637061 (4pi). The chord height of Sector {C} is √1.5 (1.224744871). Its area is 9.424777961 (3pi). The total amount of area lying outside the Orange Isosceles Triangle (A + B + C) = 34.55751919 (11pi). The hemisphere's total surface area 37.69911184 (12pi) - 34.55751919 (11pi) = the area bound by the isosceles triangle {X} 3.141592654; which is pi exactly.
Since the hemisphere is 12pi & is divided into thirds, the area of Sector {A} is 12.56637061 (4pi). The area of Sector {B} is also one third of the hemisphere's total surface area = 12.56637061 (4pi). The chord height of Sector {C} is √1.5 (1.224744871). Its area is 9.424777961 (3pi). The total amount of area lying outside the Orange Isosceles Triangle (A + B + C) = 34.55751919 (11pi). The hemisphere's total surface area 37.69911184 (12pi) - 34.55751919 (11pi) = the area bound by the isosceles triangle {X} 3.141592654; which is pi exactly.
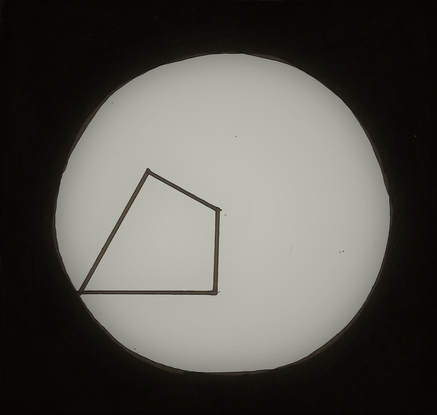
Here, I've isolated the Pink Kite-Shaped Tetragon & have divided the hemisphere's surface into thirds. Hence, the surface area of each third is equal to 12.56637061 (4pi). The area of Sector {A} is 4pi & the area of Sector {B} is also 4pi. The chord height of Sectors {C & D} is √1.5 (1.224744871). Each sector has an area of 4.71238898 (1.5pi). The combined area of (A + B + C + D) = 34.55751919 (11pi). Subtracting that sum from the hemisphere's total surface area = the area bound by the quadrilateral {X}; which is pi exactly.
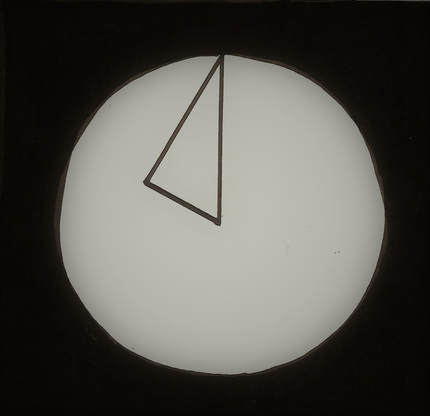
Here, I've isolated the little Yellow Triangle & increased the size of the hemisphere by six; which resembles a typical pie chart. The hemisphere's total area is 75.39822369 (24pi). Each of the six "slices" therefore, are equal to 12.56637061 (4pi). The combined area of (A + B + C + D + E) is 62.83185307 (20pi). The chord height of Sector {F} is √3 (1.732050808). Hence, its area is 9.424777961; (3pi). The combined area of all the Sectors lying outside the small yellow triangle is 72.25663103; or (23pi). Subtracting that amount from the hemisphere's total surface area leaves an area encompassed by the triangle {X} at 3.141592654... which is pi exactly.
Constructing a Rectangle with an area exactly equal to Pi
I have recently discovered an elegant method for constructing a rectangle that, when embedded in a certain hemisphere, will encompass a curved surface area exactly equal to pi. The procedure is outlined below:
I have recently discovered an elegant method for constructing a rectangle that, when embedded in a certain hemisphere, will encompass a curved surface area exactly equal to pi. The procedure is outlined below:

(1) I begin the demonstration with a typical set of nested squares & circles. This diagram is very easy to construct with unmarked compass & straightedge, so I won’t bother with extraneous details. Although measurement is not used to construct these figures, it is obviously required for the proof. Therefore, I will treat the innermost Green Circle as a Unit Circle; (Radius = 1, Area = Pi). The outer Blue Circle is twice the size of the Green Circle; (Radius = √2, Area = 2Pi). Also, you will notice that I’ve labeled key intersecting points with the letters A-E, and made two line extensions; one in the center & one at the bottom. These will come into play in succeeding diagrams.

(2) Draw an Orange line segment from point {B} to point {C}. According to the Pythagorean Theorem this line segment would have a length of √3 = (1.732050808).
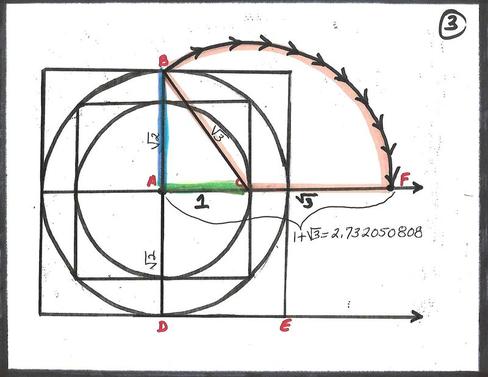
(3) Anchor the compass at Point {C}, extend it out to Point {B}, and draw the Orange Arc clockwise until it intersects the center line extension. Assign the letter {F} to the point of intersection as shown. Line segment {AF} is equal to (1 + √3) = 2.732050808.
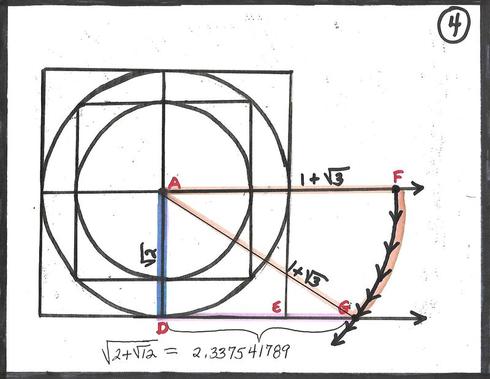
(4) Anchor the compass on Point {A}, extend it out to Point {F}, and sweep the Orange Arc down to the bottom line extension. Label the point of intersection with the letter {G} as shown. Using the Pythagorean Theorem we find that the Pink line segment {DG} is equal to (2.337541789). This will ultimately be the diameter of my final circle.
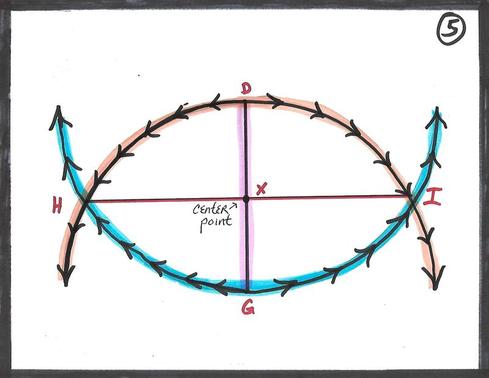
(5) I’ve erased everything to reduce clutter, and simply begin with the Pink line segment {DG} created in the preceding frame. It is situated vertically here so the drawings will fit on the page. Here, we need to bisect the Pink segment to ascertain the radius of our circle. Anchor the compass on Point {D}, extend it out to Point {G}, and sweep the Blue arc in both directions as shown. Anchor the compass on point {G}, extend it out to point {D}, and sweep the Orange arc in both directions. Label the two points of intersection with the letters {H} and {I} respectively. Draw a straight line from point {H} to point {I}… this will bisect the Pink line perfectly. Label the center point with letter {X}.
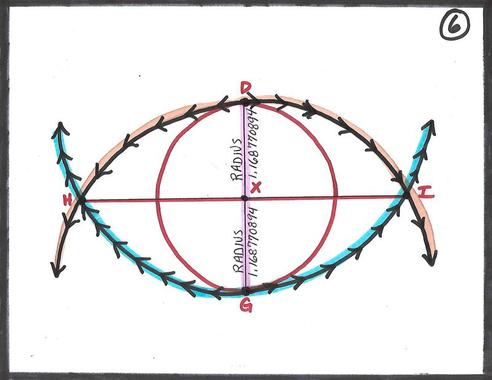
(6). Using line segment {XD} as the radius, complete the Red Circle.
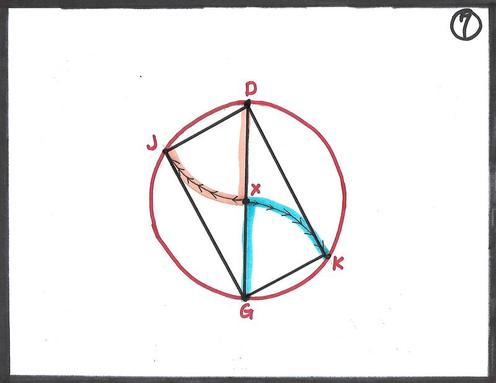
(7) Now, we have the Red Circle, with a diameter {DG}, and a center point {X}. I will now complete the inscribed rectangle. Anchor the compass on Point {G}, extend it out to Point {X}, and sweep the blue arc such that it intersects the circumference of the Red Circle; label that point with the letter {K} accordingly. Next, anchor the compass on point {D}, extend it out to point {X}, and sweep the orange arc in a similar way. Label the point of intersection with the letter {J} as illustrated. Join all four points {J-D-K-G} to complete the inscribed rectangle.
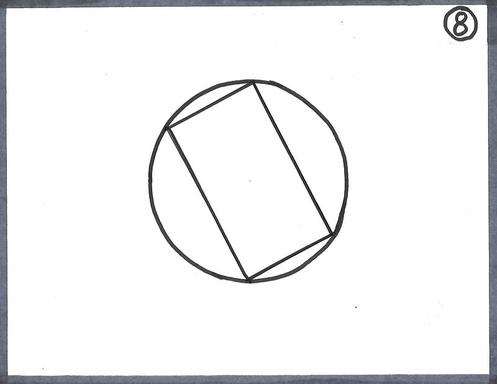
(8) This is the completed diagram. Considering we began with a Unit Circle, the following calculations will result:
Circle Dimensions: Diameter = 2.337541789
Circumference = 7.343604112
Radius = 1.168770894
Radius² = 1.366025404
Area of flat equator circle= 4.29149537
Rectangle Dimensions: Short side is the same length as the circle's radius = 1.168770894
Long side = (Short side X √3) = 2.024370572
Area = (Radius² + 1) = 2.366025404
Circle Dimensions: Diameter = 2.337541789
Circumference = 7.343604112
Radius = 1.168770894
Radius² = 1.366025404
Area of flat equator circle= 4.29149537
Rectangle Dimensions: Short side is the same length as the circle's radius = 1.168770894
Long side = (Short side X √3) = 2.024370572
Area = (Radius² + 1) = 2.366025404

Change in Manifold
(9) Just as I did above, I will now change the surface of the red circle to a smooth Riemannian Manifold of positive Ricci Curvature & exemplify that change by darkening the background with a black magic marker. Please note: The diagram itself has not changed at all; in fact, this is a photocopy.
To properly understand what I've done, imagine you walk outside in the middle of the night & gaze up at the full moon; which is actually a hemisphere; exactly 1/2 of the moon. But what you see in the night sky is not what you'd typically expect to find... instead, the image appearing in the sky is exactly like the one appearing here on your screen... a flat white circle with an inscribed rectangle. Now, imagine you take a Polaroid camera outside & snap a picture of the moon... and you take it inside, lay it on the table, measure the rectangle, and find that your measurements correspond exactly with those in this demonstration. In that case, the total amount of curved surface area enclosed by this rectangle on the lunar hemisphere would be exactly equal to pi. (See below for this rectangle's dimensions).
Like I said before, it might be easier to visualize what I’m talking about if you think of this as a hemispherical lump of cookie dough; with the flat side lying on a table. You then take a cookie cutter with dimensions identical to that of the rectangle, center it perfectly over the hemisphere, and press it down into its surface until it reaches the table. You will find that the cutter has sliced off four half-caps from the lump; two large ones & two smaller ones. When you take the sum of their surface area & subtract that from the hemisphere’s total surface area, you will find that what is left over (i.e., the rectangular space) is pi exactly.
(9) Just as I did above, I will now change the surface of the red circle to a smooth Riemannian Manifold of positive Ricci Curvature & exemplify that change by darkening the background with a black magic marker. Please note: The diagram itself has not changed at all; in fact, this is a photocopy.
To properly understand what I've done, imagine you walk outside in the middle of the night & gaze up at the full moon; which is actually a hemisphere; exactly 1/2 of the moon. But what you see in the night sky is not what you'd typically expect to find... instead, the image appearing in the sky is exactly like the one appearing here on your screen... a flat white circle with an inscribed rectangle. Now, imagine you take a Polaroid camera outside & snap a picture of the moon... and you take it inside, lay it on the table, measure the rectangle, and find that your measurements correspond exactly with those in this demonstration. In that case, the total amount of curved surface area enclosed by this rectangle on the lunar hemisphere would be exactly equal to pi. (See below for this rectangle's dimensions).
Like I said before, it might be easier to visualize what I’m talking about if you think of this as a hemispherical lump of cookie dough; with the flat side lying on a table. You then take a cookie cutter with dimensions identical to that of the rectangle, center it perfectly over the hemisphere, and press it down into its surface until it reaches the table. You will find that the cutter has sliced off four half-caps from the lump; two large ones & two smaller ones. When you take the sum of their surface area & subtract that from the hemisphere’s total surface area, you will find that what is left over (i.e., the rectangular space) is pi exactly.

Rectangle Dimensions: Since this is a hemisphere, as opposed to a flat circle, its area has now doubled in size to 8.582990739. The chord height of each of the two short sides {A & B} is .156585608. Therefore, the area of each = .574951357. The chord height for each of the two long sides is 1/2 of the radius; (.584385447). Hence, the area of each side is 2.145747686. The sum of all four areas lying outside the rectangle {A +B + C +D} = 5.441398086; which is pi times √3. Subtracting that amount from the hemisphere's total surface area = the amount of area enclosed by the rectangle; which is pi exactly.
Oddly, the sum of sectors {A + B + C + D} is exactly equal in area to the circumference of the smaller inscribed circle in my initial base diagram above. I'm not sure if this connection has any significance.
Oddly, the sum of sectors {A + B + C + D} is exactly equal in area to the circumference of the smaller inscribed circle in my initial base diagram above. I'm not sure if this connection has any significance.
Other Polygons derived from the Rectangle constructed above:
The rectangle created above could be split in half vertically (to create two long rectangles), or horizontally (to create two shortened rectangles), or diagonally (to create two triangles). Each of the two new shapes created would be equal to pi so long as the size of the hemisphere is doubled. If the rectangle is divided into fourths, and the hemisphere is increased by four, each of the four new rectangles will encompass an area of the hemisphere exactly equal to pi. Consult examples below.
The rectangle created above could be split in half vertically (to create two long rectangles), or horizontally (to create two shortened rectangles), or diagonally (to create two triangles). Each of the two new shapes created would be equal to pi so long as the size of the hemisphere is doubled. If the rectangle is divided into fourths, and the hemisphere is increased by four, each of the four new rectangles will encompass an area of the hemisphere exactly equal to pi. Consult examples below.
Here, I have cut the Rectangle in half on its horizontal axis & doubled the hemisphere's size.
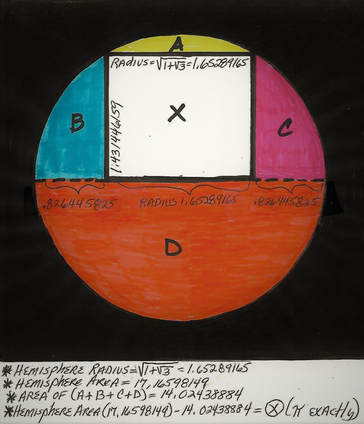
If the full moon was a perfect hemisphere & its radius was 1.65289165; and the dimensions of the quadrilateral were identical to those delineated here; and it was situated precisely as shown on this diagram, then the total amount of the hemisphere’s surface area encompassed thereby would be exactly equal to pi. The chord height of Area {A} is .221445481 (which is 2X the Form Factor Ratio Mantissa). Its area is equal to 1.149902719. The chord height of Area {B} is ½ the radius length = .826445825; its area is equal to 2.145747686. The area of {C} therefore, is also 2.145747686. The chord height of Area {D} is the Radius length (1.65289165). Its area is exactly ½ the hemisphere’s total area = 8.582990746. The sum of all four areas lying outside the quadrilateral (A + B + C + D) = 14.02438884. Subtracting that from the hemisphere’s total area will provide the amount of surface area bound by the quadrilateral {X}. 17.16598149 - 14.02438884 = 3.141592653... which is pi exactly.
If the full moon was a perfect hemisphere & its radius was 1.65289165; and the dimensions of the quadrilateral were identical to those delineated here; and it was situated precisely as shown on this diagram, then the total amount of the hemisphere’s surface area encompassed thereby would be exactly equal to pi. The chord height of Area {A} is .221445481 (which is 2X the Form Factor Ratio Mantissa). Its area is equal to 1.149902719. The chord height of Area {B} is ½ the radius length = .826445825; its area is equal to 2.145747686. The area of {C} therefore, is also 2.145747686. The chord height of Area {D} is the Radius length (1.65289165). Its area is exactly ½ the hemisphere’s total area = 8.582990746. The sum of all four areas lying outside the quadrilateral (A + B + C + D) = 14.02438884. Subtracting that from the hemisphere’s total area will provide the amount of surface area bound by the quadrilateral {X}. 17.16598149 - 14.02438884 = 3.141592653... which is pi exactly.
Here, I've cut the rectangle in half vertically & the hemisphere's area is doubled.
If the full moon was a perfect hemisphere & its radius was 1.65289165; and the dimensions of the rectangle were identical to those delineated here; and it was situated precisely as shown on this diagram, then the total amount of the hemisphere’s surface area encompassed thereby would be exactly equal to pi. The chord height of Area {A} & area {B} is the same: .221445491; (which is 2X the Form Factor Ratio mantissa). The combined area of {A} & {B} = 1.149902717. The chord height of area {C} is ½ the radius = .826445825. The area of sector {C} is 4.291495373. The chord height of section {C} is the radius length = 1.65289165. Its area is exactly ½ the hemisphere’s total area = 8.582990745. The total amount on area lying outside the rectangle (A + B+ C + D) = 14.02438884. Subtract that from the hemisphere’s total surface area (17.16598149) = pi exactly.
If the full moon was a perfect hemisphere & its radius was 1.65289165; and the dimensions of the rectangle were identical to those delineated here; and it was situated precisely as shown on this diagram, then the total amount of the hemisphere’s surface area encompassed thereby would be exactly equal to pi. The chord height of Area {A} & area {B} is the same: .221445491; (which is 2X the Form Factor Ratio mantissa). The combined area of {A} & {B} = 1.149902717. The chord height of area {C} is ½ the radius = .826445825. The area of sector {C} is 4.291495373. The chord height of section {C} is the radius length = 1.65289165. Its area is exactly ½ the hemisphere’s total area = 8.582990745. The total amount on area lying outside the rectangle (A + B+ C + D) = 14.02438884. Subtract that from the hemisphere’s total surface area (17.16598149) = pi exactly.
Here, I've cut the rectangle in half diagonally & doubled the hemisphere's area.
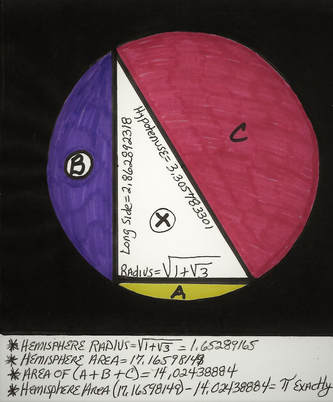
If the full moon was a perfect hemisphere & its radius was 1.65289165; and the dimensions of the 30/60/90 degree right triangle were identical to those delineated here; and it was situated precisely as shown on this diagram, then the total amount of the hemisphere’s surface area encompassed thereby would be exactly equal to pi. The chord height of Area {A} is exactly twice the Form Factor Ratio mantissa; .221445491. Hence, its area is 1.149902719. The chord height of sector {B} is ½ the radius = .826445825. Its hemispherical area is 4.291495373. The chord height of sector {C} is equal to the hemisphere’s radius length; 1.65289165. Its area is equal to exactly ½ of the hemisphere’s total surface area; 8.582990745. The total amount of surface area lying outside the triangle (A + B+ C + D) = 14.02438884. Subtract that from the hemisphere’s total surface area: (17.16598149) – 14.02438884 = pi exactly.
If the full moon was a perfect hemisphere & its radius was 1.65289165; and the dimensions of the 30/60/90 degree right triangle were identical to those delineated here; and it was situated precisely as shown on this diagram, then the total amount of the hemisphere’s surface area encompassed thereby would be exactly equal to pi. The chord height of Area {A} is exactly twice the Form Factor Ratio mantissa; .221445491. Hence, its area is 1.149902719. The chord height of sector {B} is ½ the radius = .826445825. Its hemispherical area is 4.291495373. The chord height of sector {C} is equal to the hemisphere’s radius length; 1.65289165. Its area is equal to exactly ½ of the hemisphere’s total surface area; 8.582990745. The total amount of surface area lying outside the triangle (A + B+ C + D) = 14.02438884. Subtract that from the hemisphere’s total surface area: (17.16598149) – 14.02438884 = pi exactly.
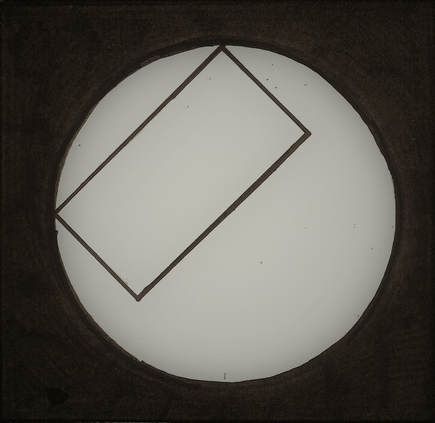
Here, I have cut the rectangle into four equal parts & increased the size of the hemisphere by four.
If the full moon was a perfect hemisphere & its radius was 2.337541789; and the dimensions of the rectangle (in flatland) were identical to those delineated here; and it was situated precisely as shown on this diagram, then the total amount of the hemisphere’s surface area encompassed thereby would be exactly equal to pi all the way into infinity. The chord height of section {A} .313171217; its area is 1.149902718. The chord height of section {B} is equal to the hemisphere’s radius; 2.337541789; its area is exactly ¼ the hemisphere’s total area = 8.582990746. The chord height of section {C} is ½ the hemisphere’s radius length; 1.168770894. The area of section {C} is, therefore, 4.291495373. The chord height of section {D} is the radius length; 2.337541789. Its area is exactly ½ the hemisphere’s total surface area; 17.16598149. Subtracting the sum of all four areas lying outside the rectangle (A + B + C + D) from the hemisphere’s total area will provide the amount of surface area bound by the rectangle {X}; which is pi exactly.
As I mentioned above with the square, any line drawn through the center of the circle (or hemisphere) will cut the initial rectangle in half such that both halves will be identical in size & shape, and both halves will be situated in precisely the same position. Since there is an unlimited number of ways to do this, there are an unlimited number of shapes that can be constructed therefrom with each half being exactly equal to pi (that is, so long as the size of the hemisphere is doubled in area).
I'm pretty sure this can be accomplished with other inscribed polygons as well (pentagons, hexagons, octagons, etc). For example: I'm pretty sure a pentagon embedded in the manifold of Sphere [A] will enclose a total amount of curved surface area equal to (2pi-3). But the math begins to get really complicated after that. Dealing with so many irrational numbers in combination with transcendental numbers makes the proof extremely difficult. This would require a fair amount of knowledge in calculus & trigonometry; which I don't have at this time & have no intention of acquiring in the near future.
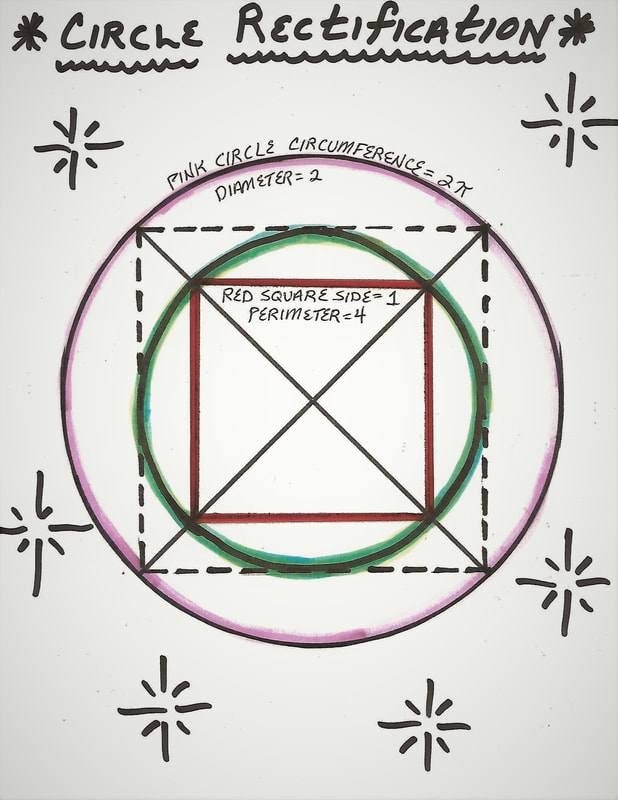
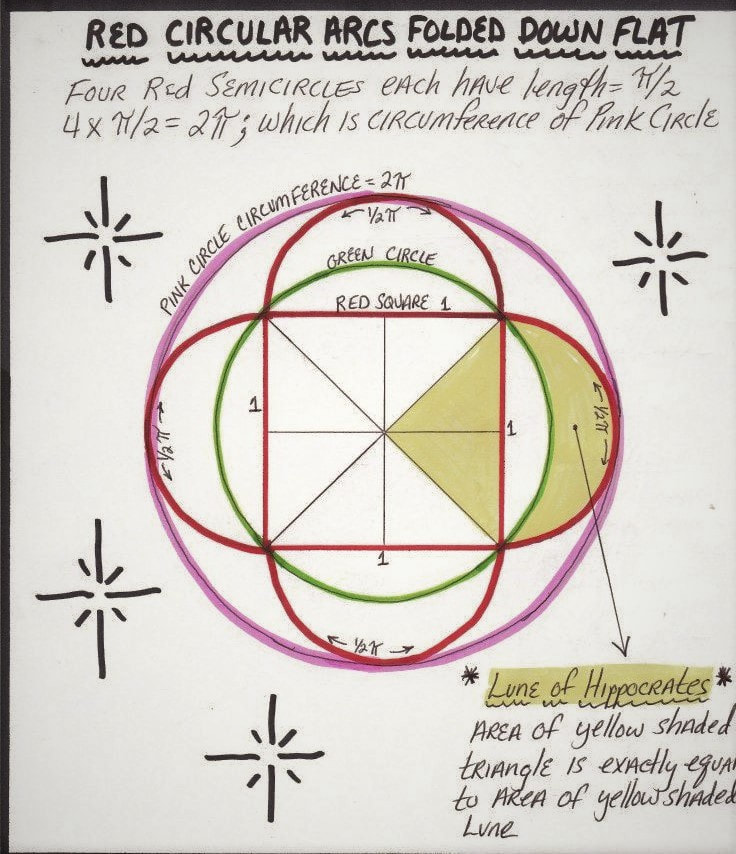
This procedure can also be used to "rectify" the circle. By that I mean it can be used to construct a square & a circle which are exactly equal in perimeter. Please refer to the next portion of this demonstration for additional details.
XXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXX
If the full moon was a perfect hemisphere & its radius was 2.337541789; and the dimensions of the rectangle (in flatland) were identical to those delineated here; and it was situated precisely as shown on this diagram, then the total amount of the hemisphere’s surface area encompassed thereby would be exactly equal to pi all the way into infinity. The chord height of section {A} .313171217; its area is 1.149902718. The chord height of section {B} is equal to the hemisphere’s radius; 2.337541789; its area is exactly ¼ the hemisphere’s total area = 8.582990746. The chord height of section {C} is ½ the hemisphere’s radius length; 1.168770894. The area of section {C} is, therefore, 4.291495373. The chord height of section {D} is the radius length; 2.337541789. Its area is exactly ½ the hemisphere’s total surface area; 17.16598149. Subtracting the sum of all four areas lying outside the rectangle (A + B + C + D) from the hemisphere’s total area will provide the amount of surface area bound by the rectangle {X}; which is pi exactly.
As I mentioned above with the square, any line drawn through the center of the circle (or hemisphere) will cut the initial rectangle in half such that both halves will be identical in size & shape, and both halves will be situated in precisely the same position. Since there is an unlimited number of ways to do this, there are an unlimited number of shapes that can be constructed therefrom with each half being exactly equal to pi (that is, so long as the size of the hemisphere is doubled in area).
I'm pretty sure this can be accomplished with other inscribed polygons as well (pentagons, hexagons, octagons, etc). For example: I'm pretty sure a pentagon embedded in the manifold of Sphere [A] will enclose a total amount of curved surface area equal to (2pi-3). But the math begins to get really complicated after that. Dealing with so many irrational numbers in combination with transcendental numbers makes the proof extremely difficult. This would require a fair amount of knowledge in calculus & trigonometry; which I don't have at this time & have no intention of acquiring in the near future.
This procedure can also be used to "rectify" the circle. By that I mean it can be used to construct a square & a circle which are exactly equal in perimeter. Please refer to the next portion of this demonstration for additional details.
XXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXXX
Centering a Square Inside a Circle with Area Equal to Pi
This portion of my demonstration is something I've been trying to accomplish ever since I began working on this problem some 15 years ago. If you look at all the polygons I've created heretofore, you will notice that they all have at least one vertex lying on the equator circle. Since my knowledge of trig & calculus is extremely limited, it was the only way I could be certain my calculations for the spherical caps were correct. That's because the formula for calculating their area is very simple & doesn't require such knowledge: chord (or cap diameter) height multiplied by the sphere's circumference = cap area. I've finally found a way to isolate the square such that it shares no points in common with the equator circle. The ten-step procedure is outlined below:
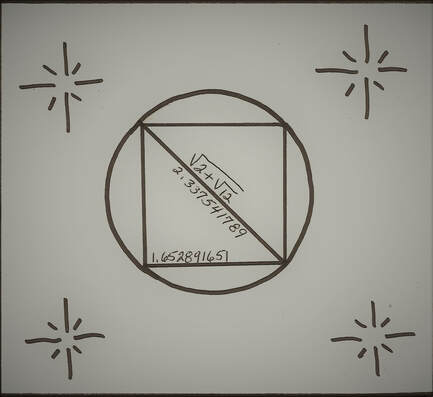
Step #1: I begin with a circle having a diameter of 2.337541789 and a radius of 1.168770894. This is the very same circle used above to create a rectangle with area equal to pi. Please consult that portion of my presentation for its construction. I then inscribe a square within that circle. The technique for accomplishing this was also illustrated earlier. In flatland this square would encompass an area equal to (1 + √3) =(2.732050808).

Step #2: I then utilize one typical phi construction by placing the compass on Point [A], extending it out to Point [B], and drawing an arc clockwise down to the square's baseline. Label the endpoint with the letter [Y] as shown.
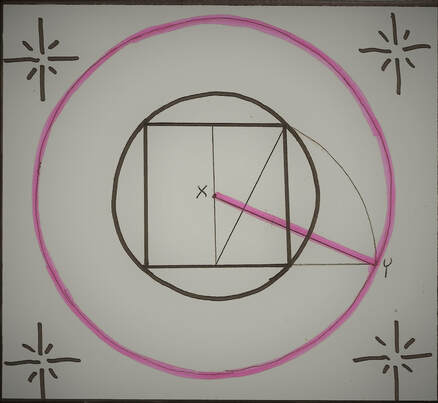
Step #3: Using line segment [XY] as a radius, draw the Pink Circle.
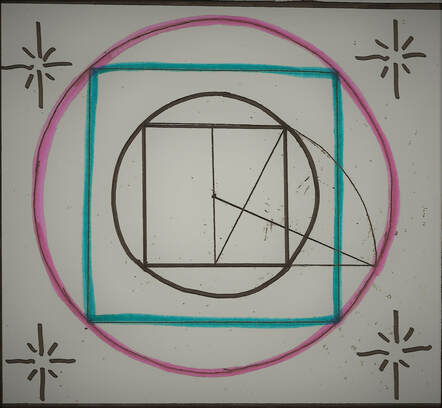
Step #4: Inscribe the Blue Square. This is easily accomplished by extending the diagonals of the Black Square outwardly to the Pink Circle & then connecting all four points of intersection.
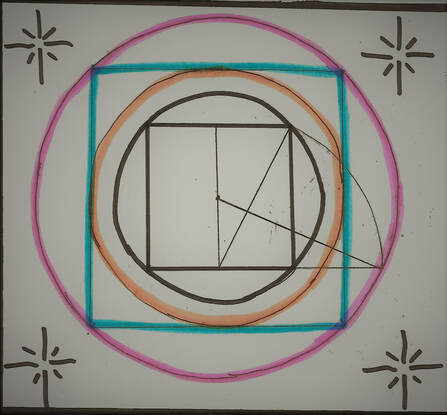
Step #5: Draw the Orange Circle inside the Blue Square.
In the next frame I will erase the Blue Square & the Black Circle to exemplify the extraordinary nature of this drawing.
In the next frame I will erase the Blue Square & the Black Circle to exemplify the extraordinary nature of this drawing.

Step #6: This frame demonstrates a unique set of circumstances in that it brings together four ubiquitous ratios in the same diagram; Pi (3.1415926), the Golden Ratio (1.6180339), the Silver Ratio (1 + √2 = 2.4142135), and (1 + √3 = 2.7320508). The Black Central Square (if it was one face of a cube) would perfectly fit inside the Orange Circle... that is, if it were a sphere... and all eight corners of that cube would come into contact with the Orange Sphere's surface at 8 equidistant points. The Pink Circle is required to create the Orange Circle & its circumference is in Golden Ratio with the sides of the Black Square. The circumference of the Orange Circle is in Silver Ratio with the sides of the Black Square. If that Black Square (with a flat area of 1 + √3) were to be superimposed upon the Orange Sphere (as illustrated in the diagram below), it would encompass an area exactly equal to pi; ad infinitum.
Correction: Each side of the Black Square [Segments (BB); not (AB)] are in Golden Ratio with Segments (BD).
Correction: Each side of the Black Square [Segments (BB); not (AB)] are in Golden Ratio with Segments (BD).
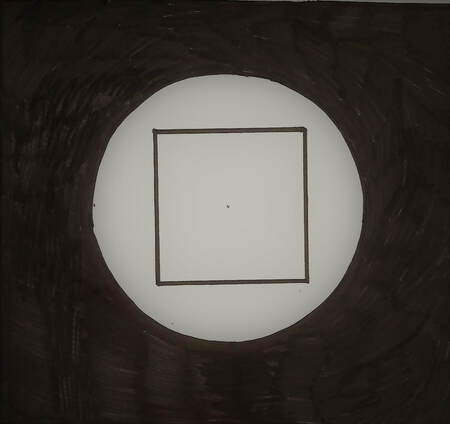
Step #7: Now I erase everything except the Orange Circle & the Black Square. As in previous diagrams I have changed the surface to a smooth Riemannian Manifold of positive curvature & exemplified that by darkening the background with a black magic marker. Otherwise, nothing else has changed; both shapes retain their original size. As noted earlier, the best way to envision this is to imagine walking outside in the middle of the night & seeing this square on the surface of a full moon. Even though everything is curved, that cannot be detected from this angle and it appears to be a flat square centered within a flat white disc.
So what we really have then is a sphere with what appears to be a perfect square etched into its surface. As indicated above, this square actually represents one side of the cube that would perfectly fit inside that sphere; with all eight corners coming into contact with the sphere's shell at eight equidistant points. Again, the observer cannot see the entire cube because it is being viewed from Blueprint Perspective.
So what we really have then is a sphere with what appears to be a perfect square etched into its surface. As indicated above, this square actually represents one side of the cube that would perfectly fit inside that sphere; with all eight corners coming into contact with the sphere's shell at eight equidistant points. Again, the observer cannot see the entire cube because it is being viewed from Blueprint Perspective.

Step #8: Here's where things get somewhat tricky. All five colored line segments are identical in length to the cube face diagonal; colored red; (2.337541789). These colored lines represent the diameters of five geodesic circles on the surface of the sphere; with the Red one representing the diameter of the thin black circle around the Black Square at the top of the sphere. These circles are drawn around each of the six faces of the cube; the diameter of the sixth circle cannot be seen since it is on the opposite side of the globe. The four geodesics around the sides of the cube (Green, Pink, Blue, & Orange) appear to be straight lines due to the observer's vantage point; but they are actually circles upon the sphere's surface; much like four intersecting paper plates standing on edge. To get a better idea of what these four geodesics look like & how they relate to the subject at hand, consult the Four Ellipse Venn Diagram on the next frame.

Step #9: Obviously, this diagram is not drawn according to scale, but that's not really important here. It's sole purpose is to exemplify what the four geodesics around the sides of the cube would look like if the sphere was transparent. As you can see, they are actually circles on the sphere's surface; not straight lines. There are also two more circles around the top & bottom of the cube, but I've eliminated them here to reduce clutter.
Interestingly, if you add the area of all six (flat) circles around the six faces of the cube, the sum is exactly equal to the surface area of the circumscribing sphere. This unique phenomenon can be used to calculate the area of any sphere around a cube.
Interestingly, if you add the area of all six (flat) circles around the six faces of the cube, the sum is exactly equal to the surface area of the circumscribing sphere. This unique phenomenon can be used to calculate the area of any sphere around a cube.

Step #10: If we imagine the six circles around each of the cube faces as spherical caps we find that they "overlap" at 12 locations; eight can be seen here (shaded in black); four around the center square & four around the equator. There are four more on the opposite side of the globe. Although they appear to be different in size & shape, they are actually identical. Again, this is due to the observer's viewpoint. This is pretty hard to visualize, but is easily demonstrated by drawing this out on a three-dimensional sphere.
Obviously, the area of all six caps is larger than the sphere itself due to the overlap. If we subtract the total amount of sphere area from the amount of area encompassed by the six caps, we will obtain the amount of area in all 12 overlaps. Dividing that by 12 will provide the amount of area in each overlap. Multiplying that by 4 will give us the amount of overlap on each cap. Subtracting that amount from the cap area will give us the amount of area encompassed by the square; which is pi exactly.
Obviously, the area of all six caps is larger than the sphere itself due to the overlap. If we subtract the total amount of sphere area from the amount of area encompassed by the six caps, we will obtain the amount of area in all 12 overlaps. Dividing that by 12 will provide the amount of area in each overlap. Multiplying that by 4 will give us the amount of overlap on each cap. Subtracting that amount from the cap area will give us the amount of area encompassed by the square; which is pi exactly.
Doubtless, additional polygons could be created from this diagram with each one encompassing an area on the hemisphere exactly equal to pi. This could easily be accomplished by cutting this square in half and doubling the size of the sphere accordingly; as demonstrated in previous diagrams. Below are two examples of shapes that would encompass an area exactly equal to pi by slicing the square in half & doubling the size of the sphere to 34.33196299.

Also, as mentioned above, any line drawn through the center of the circle (or hemisphere) will cut this square in half such that both halves will be identical in size & shape, and both halves will be situated in precisely the same position on the hemisphere. Hence, both halves will encompass the same amount of surface area on the sphere. Since there is an unlimited number of ways to do this, there are an unlimited number of shapes that can be constructed therefrom with each half being exactly equal to pi; that is, so long as the size of the sphere is doubled in size. Here is just one example.
Other polygons may be derived from this diagram that encompass an area exactly equal to pi by dividing the square into four identical shapes & increasing the size of the sphere by four to 68.66392597. This is easily achieved by connecting the diagonals or midpoints of the square. So long as each polygon is the same size & holds the same position on the sphere, each will have the same amount of surface area. (See the two examples below). Both of the two shapes below could be cut in half once again (creating a small right triangle on the left & a small isosceles triangle on the right); with both halves encompassing an area on the sphere exactly equal to pi; that is so long as the sphere's size was increased by eight.
Part Two: Circle Rectification
Typically, when mathematicians speak of "squaring the circle" they are referring to the classic problem of creating a square and a circle with unmarked compass & straightedge that are equal in area. Heretofore, this problem has been deemed "impossible" by most respectable mathematicians due to the transcendental nature of pi. When speaking of "circle rectification" they are usually referring to creating a square & a circle with the same perimeter. This problem has also has been deemed "impossible" for the same reason they claim it is "impossible" to square the circle in area. But this is fairly easy to accomplish & demonstrate using the same technique I used to square the circle above.
Typically, when mathematicians speak of "squaring the circle" they are referring to the classic problem of creating a square and a circle with unmarked compass & straightedge that are equal in area. Heretofore, this problem has been deemed "impossible" by most respectable mathematicians due to the transcendental nature of pi. When speaking of "circle rectification" they are usually referring to creating a square & a circle with the same perimeter. This problem has also has been deemed "impossible" for the same reason they claim it is "impossible" to square the circle in area. But this is fairly easy to accomplish & demonstrate using the same technique I used to square the circle above.
|
Diagram #1: Here, we have a typical set of nested squares & circles. This is very easy to produce with unmarked compass & straightedge so I won't go into the construction thereof.
Considering the red square as a unit square, the following dimensions will result: Red Square: Side = 1, Perimeter = 4. Green Circle: Diameter = √2, Circumference = 4.442882938. Pink Circle: Diameter = 2, Circumference = (2pi) 6.283185307. In forthcoming diagrams I will demonstrate how the perimeter of the red square can be exactly equal in length to the circumference of the pink circle. Diagram #2: Here I've done the same thing that I did in Part One of this presentation: I have isolated the red square & the green circle, but I have erased the colors to enhance the effect. I've also changed the manifold to one of positive curvature & exemplified that by darkening the background with a black magic marker. Let me remind you that the diagram itself has not changed... in fact, this is a photocopy. The best way to understand what I'm talking about is to imagine this as a full moon; which is actually a hemisphere; exactly one-half of the moon. By definition this is a two-dimensional surface: From this angle curvature is not detectable & every single point on its entire surface is visible. Diagram #3: Blueprint Perspective: The top diagram is what this looks like from from directly above. The red square is perfectly centered inside the green hemispherical equator circle. The bottom diagram shows what this would look like from the side. Here, we clearly see that the sides of the red square are actually curved. They are precisely one-half of a circle. The diameters of these circles are equal in length to the sides of the red square, which is 1. Therefore, the actual length of each red arc is exactly 1/2 pi. All four added together are exactly equal to the circumference length of the pink circle above (2pi). Diagram #4: Here's a crude 3D model showing the sides of the square as four upswept arcs. These arcs are actually semi-circles (or half-circles) on the surface of the hemisphere. They are tangent at four equidistant points on the hemisphere's equator circle. When viewed from directly above the curvature cannot be detected; much like four paper plates set on edge; and it looks like a flat white square centered in a flat circle. The gray shaded area that would be supported by these arcs is a perfect rendition of a sail vault; from which the pendentive dome was eventually developed. This was first used in Hagia Sophia & was considered an architectural marvel. It was the first time that a square base made a graceful transition to the circular structure above; in essence, squaring the circle. This was the culmination of many years of architectural development & was preceded by corbelling, groin vaults, and polygonal domes; which I will examine more closely in upcoming diagrams. Diagram #5: Here, I've folded the red arcs down flat so their dimensions could be seen more easily. Now we state the obvious: Each Red half-circle is precisely equal in length to 1/2pi. All four would be equal to the pink circle's circumference. Conclusion: The perimeter of the Red Square, when embedded in a two-dimensional smooth Riemannian manifold of positive curvature & bound by an equator circle the size of the Green Circle, is EXACTLY EQUAL in length to the circumference of the Pink Circle. The circle is rectified via compass & straightedge. Incidentally, the famous "Lune of Hippocrates" makes an appearance here. The two yellow shaded areas (the lune & the triangle) are exactly equal in area. Although unintentional I doubt this is sheer happenstance, since all these things are interrelated. Diagram #6: Groin Vault; also known as the “Cross Vault;” for obvious reasons. In religiously oriented architecture it can be used to represent the Cross of Christ & I will exemplify that point as I proceed through the next few frames. This structure can be found most commonly in basement & ground floor levels of buildings due to its ability to support heavy payloads without the need for massive buttresses. It achieves this by directing the stresses almost vertically downward through the four corner piers. It was highly influential during the Middle Ages & was frequently employed in Gothic Cathedrals. Oftentimes, it was used to construct long corridors by aligning several of them in a row. When compared to Diagrams 1-5 the relationship between this structure & the topic at hand is self-evident. The four red arcs & the Red Square show up quite nicely. The design of the Groin Vault was derived directly from a bicylinder which has been cut in half horizontally. (See the next diagram). Diagram #7: The Bicylinder is formed by two cylinders of equal diameter intersecting at right angles. The interesting thing about cylinders is that Euclidean math is applicable. As you can see from the diagram on the left, the Red Square is very prominent. When the cylinder height (or length if you prefer) is reduced to its extreme minimum as portrayed in the bottom drawing, the four openings become tangent & meet at right angles. In other words, if the height of each cylinder was equal in length to its diameter (which, in this case is 1), then it would perfectly reflect the dimensions of the Red Square & the red arcs depicted in previous frames. If you were to cut it in half horizontally, you’d have the Groin Vault illustrated in the previous frame. The sphere within which this could be circumscribed would have a diameter equal to the cylinder’s diameter length (1) multiplied by √2. In this case, the bottom bicylinder would perfectly fit inside the Green Circle; that is, of course, if it were actually a sphere. The four cylinder openings would come into contact with the surface of the sphere & trace out four perfect circles. Each Red Circle would be tangent to the adjacent one at a single point on the Green Sphere’s equator circle. They would meet at right angles & would look exactly like the previous diagrams. From directly above curvature of the arcs would not be detectable & it would look exactly like the Red Square inscribed in the Green Circle. |

Diagram #8: Steinmetz Solid: This is what it would look like if we filled the bicylinder with a substance & then allowed it to harden into a solid. The outer shell of this particular solid forms a four-sided polygonal dome; which has been used extensively in holy sites; especially the octagonal style. The Steinmetz Solid is quite remarkable in that it has perfectly SQUARE cross sections even though it was formed by the intersection of two perfectly ROUND cylinders. I knew I was on the right track when I saw that. The object is unusual; something like a queer deck of cards: It has the smallest square card on the bottom. From there the cards remain square in shape; but grow progressively larger as they approach the middle of the deck. And then they grow progressively smaller as they approach the top of the deck. Perfect circles the size of the Red Circle can be drawn around its midpoints; while perfect ellipses can be drawn around its vertices. These squares appear to steadily diminish in size until they collapse into a single point, much like the circles on a sphere in the Poincare Conjecture.
From these diagrams we can see what happens when we have two cylinders of equal diameter intersect horizontally at right angles. Now, let’s see what happens when we drive a third cylinder down vertically or perpendicularly through the center of those two cylinders.
From these diagrams we can see what happens when we have two cylinders of equal diameter intersect horizontally at right angles. Now, let’s see what happens when we drive a third cylinder down vertically or perpendicularly through the center of those two cylinders.
Diagram #9: The Tricylinder is formed by three cylinders of equal diameter intersecting at right angles. In this particular case the diameter of each cylinder would be the same length as the side length of the Red Square (1). If all three cylinders are reduced to their minimum height of 1 (as we did with the bicylinder in Diagram #7; bottom drawing), and then inscribed this object within the Green sphere, it would trace out six circles upon its surface: Four around the equator circle & one each around the North & South Poles. It basically takes on the same shape as a cube, but instead of squares on each of it’s six faces, it has circles instead. See the next frame for a 3D image.

Diagram #10: This is what the Tricylinder would look like if all three cylinders were reduced to the minimum height of 1. All six openings would be tangent to one another at four equidistant points. If we were to inscribe this object within the green sphere, it would trace out six perfect circles upon its surface; four around the equator & one each on the top & bottom. The eight "triangular" shapes at the corners are "pendentives." If we cut this object in half on its horizontal axis you will be able to see how it relates to the subject at hand.
Diagram #11: Here, we've simply cut the Tricylinder in half on it’s horizontal axis. This reflects the floorplan for Hagia Sophia (Gr. Holy Wisdom). This is a Byzantine Church located in Istanbul Turkey, formerly Constantinople. It was constructed around 530 A.D. by Emperor Justinian. It was the first time the pendentive dome was used & it was immediately recognized as an architectural marvel. It still is. Upon it's completion Justinian is said to have exclaimed: "Solomon I have outdone thee!" Obviously, he was comparing it to Solomon’s Temple and referring to the sacred geometry & great wisdom utilized in its construction.
The fact that the "footprint" of this object is identical to the floorplan of the church is inescapable. It's impossible to ascertain whether the architects already knew this & did it deliberately... or not. But there's no doubt that the design of this structure, as well as its name, were both Divinely inspired.
The fact that the "footprint" of this object is identical to the floorplan of the church is inescapable. It's impossible to ascertain whether the architects already knew this & did it deliberately... or not. But there's no doubt that the design of this structure, as well as its name, were both Divinely inspired.
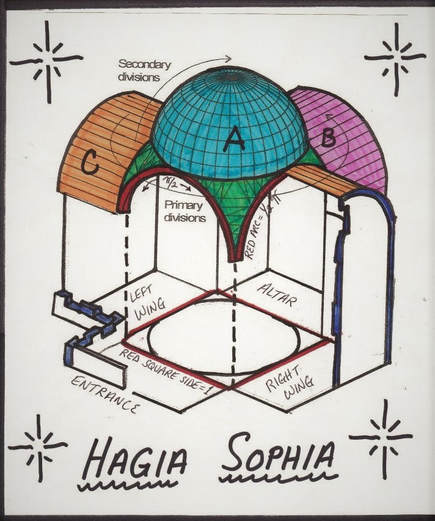
Diagram #12: Hagia Sophia: This was the seat of the Eastern Orthodox Church for nearly ten centuries. It's geometric design represents the marriage of heaven & earth; God & man through Christ Jesus. And "happy are those who are called to the Marriage Supper of the Lamb," (Rev. 19:7-9). In sacred geometry the Circle represents God & the square represents man. The circular dome makes a graceful transition to the square base below via the pendentives. These pendentives are the strange looking green “triangles” at each of the four corners. This evenly distributes the immense weight of the massive dome & channels it down through the four main columns at the four corners. Traditionally, the portraits of the four evangelists are depicted on these four pendentives: (Matthew, Mark, Luke & John). The theme is extremely symbolic. It represents God (the Circle) reconciling Himself with man (the square) by way of the pendentives (the Gospel of of our Lord Jesus Christ). As you can plainly see, the entire layout is shaped like a crucifix. Once in a while you might even encounter the phrase "square the circle" when researching this subject. It's usually employed figuratively or allegorically. But there's no doubt (at least in my mind) that it also solves this mystery in a literal sense.
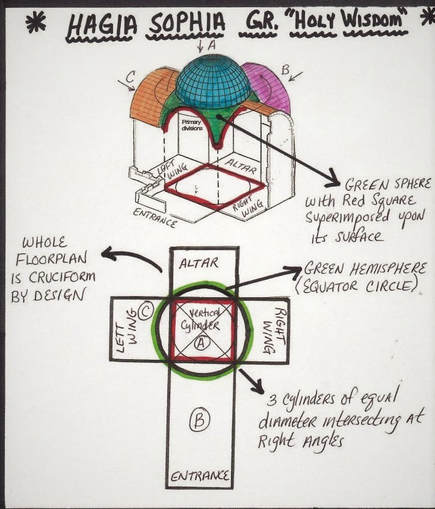
Diagram #13: Here's another look at the floorplan of Hagia Sophia. As you can see the whole thing is deliberately cruciform. Otherwise, the diagram is basically self-explanatory.
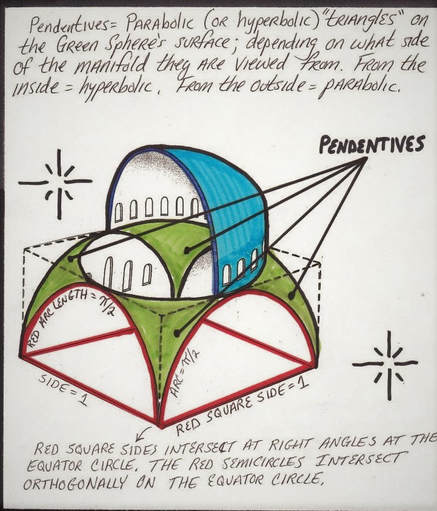
Diagram #14: Here's another look at the Pendentive Dome: The relationship between the four Red semi-circles & the four side lengths of the Red Square is very easy to see. I have figured out how to calculate it’s surface area: It is equal to the Green hemisphere’s surface area (pi) divided by the Silver Mean. Pi ÷ (1 + √2) = 1.301290318. As illustrated in Part One of this presentation, if the square (in flatland) had an area equal to the Silver Ratio, and was projected onto a positively curved surface, the resulting sail vault would enclose an area exactly equal to pi. The pendentives are the green “triangles” at each of the four corners. They can be concave or convex depending on what side of the structure you are on when viewing them. They look like deltoids (that's a three-sided hypocycloid). But they're not. I’ve figured out how to calculate their surface area too: Area of the four pendentives is equal to the total surface area of the sail vault divided by (1 + the Silver Mean). In this particular case the four pendentives would have a total surface area of .381139; with each pendentive having a surface area of .095284779.
As I indicated very early on, when I began this project I couldn’t remember even the most basic principles of geometry. I essentially started from scratch. I spent the first three years focusing on three sets of isometrically related nested squares & circles, the Divine Proportion, and the hypocycloid. At first I couldn’t figure out where the hypocycloid fit in. Some time around the beginning of my fourth year into this I met a guy who held a PhD in Mechanical Engineering. He spoke of lines on a sphere & Bingo! There’s where my hypocycloid belonged! I was very excited about this development & for the next several months my focus centered upon what I called “the square on a sphere.” As I began to approach the beginning of my fifth year into this, progress had once again slowed to a snail’s pace.
So one day, kinda outta the blue, I decided to attend Easter mass at Good Shepherd Catholic Church. I hadn't been to mass in over 30 years. I arrived late so I stood in the very back. For whatever reason I glanced up at the massive dome & there it was; plain as day; my square on a sphere! I was utterly flabbergasted. Needless to say, as soon as mass was over I rushed home to see what I could find out about this on the Internet. It wasn't long before I found that my square on a sphere had a name; it was called a sail vault. I examined hundreds of images of this unique structure & found that if I viewed it from a great enough height it would resemble a perfect inscribed square. And if I were to lay on my back in the middle of the Church & stare straight up at it, I’d see my hypocycloid staring right back down on me. Compare Diagram #15 (the hypocycloid) with Diagram #16. This event was a huge turning point in my research. My mission was back on track. Information began pouring in faster than I could process it. I began inscribing Bicylinders & Tricylinders inside spheres. I found that these objects carve out perfect circles on the sphere's surface identical to those we've been discussing. When viewing the inscribed Bicylinder from directly above, the curvature of each of its four openings could not be detected; much like looking at four paper plates sideways; and the four tangent circles appeared to be a perfect square (just like the Red Square in previous diagrams). Later, I would study their respective Steinmetz Solids; which led me to investigate groin vaults & polygonal domes. I then began plucking different squares from my three sets of isometric drawings & projecting them onto different spheres. And the Golden Mean just fell outta the sky. Seriously. I wasn’t even looking for this or trying to square the circle from this angle. I found that certain squares were in Golden Ratio with certain circles and before I knew it, the method for squaring the circle depicted in Part Three of this demonstration was right there on the sheet of paper lying before me. I know it may sound incredible, but it’s almost as if I didn’t even draw it myself.
Ya know: It’s funny: It seems like I learned nearly everything I know about this problem in reverse. It's as if I slipped in through the back door. It was like the popular game show Jeopardy; where the contestants are given the answer & then challenged to frame the appropriate question to match it. You'd think I had started with the pendentive dome and then worked my way backwards; especially considering the fact that it represents the squaring of the circle and/or the Incarnation of the Son of God. (This is what surprises me the most. Why hasn't somebody already done this?) Anyway, as unbelievable as it may sound, I had absolutely no idea that this math problem had such a deeply spiritual side to it. As far as I was concerned the only spiritual aspect of it was that a guy asserted God Himself couldn’t do it. It wasn’t until that Easter morning a couple years ago that I realized it had so many spiritual implications.
As I indicated very early on, when I began this project I couldn’t remember even the most basic principles of geometry. I essentially started from scratch. I spent the first three years focusing on three sets of isometrically related nested squares & circles, the Divine Proportion, and the hypocycloid. At first I couldn’t figure out where the hypocycloid fit in. Some time around the beginning of my fourth year into this I met a guy who held a PhD in Mechanical Engineering. He spoke of lines on a sphere & Bingo! There’s where my hypocycloid belonged! I was very excited about this development & for the next several months my focus centered upon what I called “the square on a sphere.” As I began to approach the beginning of my fifth year into this, progress had once again slowed to a snail’s pace.
So one day, kinda outta the blue, I decided to attend Easter mass at Good Shepherd Catholic Church. I hadn't been to mass in over 30 years. I arrived late so I stood in the very back. For whatever reason I glanced up at the massive dome & there it was; plain as day; my square on a sphere! I was utterly flabbergasted. Needless to say, as soon as mass was over I rushed home to see what I could find out about this on the Internet. It wasn't long before I found that my square on a sphere had a name; it was called a sail vault. I examined hundreds of images of this unique structure & found that if I viewed it from a great enough height it would resemble a perfect inscribed square. And if I were to lay on my back in the middle of the Church & stare straight up at it, I’d see my hypocycloid staring right back down on me. Compare Diagram #15 (the hypocycloid) with Diagram #16. This event was a huge turning point in my research. My mission was back on track. Information began pouring in faster than I could process it. I began inscribing Bicylinders & Tricylinders inside spheres. I found that these objects carve out perfect circles on the sphere's surface identical to those we've been discussing. When viewing the inscribed Bicylinder from directly above, the curvature of each of its four openings could not be detected; much like looking at four paper plates sideways; and the four tangent circles appeared to be a perfect square (just like the Red Square in previous diagrams). Later, I would study their respective Steinmetz Solids; which led me to investigate groin vaults & polygonal domes. I then began plucking different squares from my three sets of isometric drawings & projecting them onto different spheres. And the Golden Mean just fell outta the sky. Seriously. I wasn’t even looking for this or trying to square the circle from this angle. I found that certain squares were in Golden Ratio with certain circles and before I knew it, the method for squaring the circle depicted in Part Three of this demonstration was right there on the sheet of paper lying before me. I know it may sound incredible, but it’s almost as if I didn’t even draw it myself.
Ya know: It’s funny: It seems like I learned nearly everything I know about this problem in reverse. It's as if I slipped in through the back door. It was like the popular game show Jeopardy; where the contestants are given the answer & then challenged to frame the appropriate question to match it. You'd think I had started with the pendentive dome and then worked my way backwards; especially considering the fact that it represents the squaring of the circle and/or the Incarnation of the Son of God. (This is what surprises me the most. Why hasn't somebody already done this?) Anyway, as unbelievable as it may sound, I had absolutely no idea that this math problem had such a deeply spiritual side to it. As far as I was concerned the only spiritual aspect of it was that a guy asserted God Himself couldn’t do it. It wasn’t until that Easter morning a couple years ago that I realized it had so many spiritual implications.

Diagram #15: Hypocycloid: Here is a diagram of a unique kind of hypocycloid; also known as an astroid (not asteroid). This, along with the Golden Mean, was the center of my attention for the first several years. This particular hypocycloid totally fascinated me & opened many doors for me. When a circle is inscribed within a square, the hypocycloid consists of all that area lying outside the circle but inside the square. It is the four curved shapes at the four corners. When folded inwardly to the center they come together to produce the single shape that is the hypocycloid. I knew it was important right away because:
(1). It had many properties of a square, yet it was comprised of nothing but curves.
(2). The length of it's perimeter was exactly equal to the length of the circumference of the inscribed circle.
(3). Although comprised solely of curves its area could be added to other shapes comprised solely of curves to attain an area exactly equal to a shape comprised solely of straight lines; namely a square. For example: One Hypocycloid + One Circle = One Square. Also, Two Hypocycloids + Four Vesica Pisces = One Square.
Due to its name (Gr. astroid; "Star"), I used to refer to it as the Star of David. But since it became the key to unlocking this mystery, I now call it the Key of David. For the first few years I couldn't figure out where the hypocycloid fit in. But now I know. It can be used to rectify the circle exactly. I’ve also heard that the hypocycloid squares the circle hyperbolically in the Poincare Disk. That certainly wouldn’t surprise me. M.C. Escher offers a superb rendition of it in his artwork (Circle Limit III). Please turn to the next diagram to see how this shape relates to the subject at hand.
(1). It had many properties of a square, yet it was comprised of nothing but curves.
(2). The length of it's perimeter was exactly equal to the length of the circumference of the inscribed circle.
(3). Although comprised solely of curves its area could be added to other shapes comprised solely of curves to attain an area exactly equal to a shape comprised solely of straight lines; namely a square. For example: One Hypocycloid + One Circle = One Square. Also, Two Hypocycloids + Four Vesica Pisces = One Square.
Due to its name (Gr. astroid; "Star"), I used to refer to it as the Star of David. But since it became the key to unlocking this mystery, I now call it the Key of David. For the first few years I couldn't figure out where the hypocycloid fit in. But now I know. It can be used to rectify the circle exactly. I’ve also heard that the hypocycloid squares the circle hyperbolically in the Poincare Disk. That certainly wouldn’t surprise me. M.C. Escher offers a superb rendition of it in his artwork (Circle Limit III). Please turn to the next diagram to see how this shape relates to the subject at hand.

Diagram #16: Here is an image of a pendentive dome viewed from inside the Church. (In this case it is the Church of the Holy Sepulchre in Jerusalem). If you look up at it from directly below the hypocycloid comes into full view. If you view the same shape from directly above at great enough height, say, from God’s point of view, it resembles a perfect square.

Diagram #17: This is what the equilateral triangle would look like. It divides the hemisphere's surface into four equal sectors; three outside the triangle & the area enclosed within. If the hemisphere's radius is √2, the area of the hemisphere would be 4pi, and each of these four sectors would be exactly equal to pi.
Part Three:
Squaring the Circle with remarkable accuracy using the Golden Ratio
This portion of my presentation is a bit different from the rest in that the material introduced here does not square the circle with exact precision; but it does come remarkably close. Most of the work presented here has already been featured on Gary Meisner’s excellent website on the Golden Ratio: www.goldennumber.net/squaring-the-circle/ But I’ve decided to include it here at the end of my presentation for two reasons: (1). It yields a remarkably tight approximation for pi; accurate to four decimal points. (2). Like I said in Part Two (Diagram #14), I was working on this problem from a totally different angle, projecting squares onto spheres, when the Golden Ratio just fell outta the sky. I was beginning to lose hope & this was like a gift from God; restoring my faith & increasing incentive to continue my work even though a solution seemed bleak. I really needed the encouragement at this time, for I did not achieve the final solution for about another year after this discovery.
Unbelievably, I cannot find anything resembling the diagrams provided here. The math is all over the place; especially in religious sites, Gothic Cathedrals, and analogies dealing with the Great Pyramids of Egypt. But drawings are downright scarce. I suppose that's because many mathematicians have abandoned the drawing board in favor of the calculator. It seems that if a problem can be worked out mathematically, then they conclude it can be drawn geometrically. How to actually accomplish the task is rarely demonstrated. This is sad because many ancient problems were solved the other way around. They were solved by logic & then proven mathematically later on. This was often achieved using the age-old childlike method of paper-folding. This remains a viable & powerful tool in geometry today. For example, Hippocrates demonstrated that certain lunes could be squared even though the feat should be just as mathematically impossible as squaring the entire circle; for both shapes are inextricably connected to the same transcendental number (pi). Since then several other lunes have been squared in much the same way. These things were not accomplished mathematically & then proven logically, but vice versa. If these problems were approached with only a calculator in hand they may never have been solved.
Ramanujan devised algebraic equations that have approximated pi to several decimal places. Incidentally, he also discovered a formula which produces the same close estimation created by the Golden Mean: (9/5 + √9/5) = 3.14164. Who knows how he came up with that? Anyway, he ultimately developed an "infinite series" for pi which, when plotted into a supercomputer, is capable of calculating pi to billions or even trillions of decimal places. It is worthy to note that he continued to work on this problem even after 1882, when Ferdinand von Lindemann proved that pi was transcendental. To me, this shows that he apparently did not consider the matter closed as to whether or not the circle could be squared. He's not the only one either. There have been a few others.
The earliest efforts to square the circle often centered around the use of inscribed polygons: The idea being that the more sides one adds to the polygon the more area of the circle it would occupy. The hope was that eventually the entire circle would be filled in. For example: An inscribed pentagon would occupy more space within the circle than an inscribed square, and an inscribed hexagon would occupy more space that an inscribed pentagon, and an inscribed octagon would occupy more space within the circle than an inscribed hexagon, and so on. If proven it would also prove that the circle could be squared since polygons can be squared.
To create a square & a circle as close as the solution presented here, one would require the use of inscribed polygons of multiple facets. Archimedes used a 96-gon to set upper & lower bounds for pi of 3.142857 & 3.140845 respectively. Ptolemy used a 360-gon to obtain a value of 3.141666. That’s a super close approximation. But the one generated by these diagrams is even better than that, (3.14164). Huygens defined this number as his upper limit for pi using a square & an octagon. Liu Hui obtained the exact same ratio by inscribing a polygon of 3,072 sides back in the 3rd Century. And Aryabhatta, some time around 500 AD, used a 384-gon to obtain the same close estimate. Imagine trying to draw any one of those with compass & straightedge! I doubt they ever drew any such thing though. Like I said, mathematicians rarely drew these problems out on a sheet of paper in a literal sense.
I will lay out all the diagrams necessary to accomplish this task in detail. You can simply skip these diagrams if you wish & consult the slideshow created for me by Mr. Meisner on the aforementioned website. Here is a link to that slideshow which exemplifies all the steps in quick succession. www.goldennumber.net/wp-content/uploads/2012/05/ricci-squaring-the-circle1.gif
Squaring the Circle with remarkable accuracy using the Golden Ratio
This portion of my presentation is a bit different from the rest in that the material introduced here does not square the circle with exact precision; but it does come remarkably close. Most of the work presented here has already been featured on Gary Meisner’s excellent website on the Golden Ratio: www.goldennumber.net/squaring-the-circle/ But I’ve decided to include it here at the end of my presentation for two reasons: (1). It yields a remarkably tight approximation for pi; accurate to four decimal points. (2). Like I said in Part Two (Diagram #14), I was working on this problem from a totally different angle, projecting squares onto spheres, when the Golden Ratio just fell outta the sky. I was beginning to lose hope & this was like a gift from God; restoring my faith & increasing incentive to continue my work even though a solution seemed bleak. I really needed the encouragement at this time, for I did not achieve the final solution for about another year after this discovery.
Unbelievably, I cannot find anything resembling the diagrams provided here. The math is all over the place; especially in religious sites, Gothic Cathedrals, and analogies dealing with the Great Pyramids of Egypt. But drawings are downright scarce. I suppose that's because many mathematicians have abandoned the drawing board in favor of the calculator. It seems that if a problem can be worked out mathematically, then they conclude it can be drawn geometrically. How to actually accomplish the task is rarely demonstrated. This is sad because many ancient problems were solved the other way around. They were solved by logic & then proven mathematically later on. This was often achieved using the age-old childlike method of paper-folding. This remains a viable & powerful tool in geometry today. For example, Hippocrates demonstrated that certain lunes could be squared even though the feat should be just as mathematically impossible as squaring the entire circle; for both shapes are inextricably connected to the same transcendental number (pi). Since then several other lunes have been squared in much the same way. These things were not accomplished mathematically & then proven logically, but vice versa. If these problems were approached with only a calculator in hand they may never have been solved.
Ramanujan devised algebraic equations that have approximated pi to several decimal places. Incidentally, he also discovered a formula which produces the same close estimation created by the Golden Mean: (9/5 + √9/5) = 3.14164. Who knows how he came up with that? Anyway, he ultimately developed an "infinite series" for pi which, when plotted into a supercomputer, is capable of calculating pi to billions or even trillions of decimal places. It is worthy to note that he continued to work on this problem even after 1882, when Ferdinand von Lindemann proved that pi was transcendental. To me, this shows that he apparently did not consider the matter closed as to whether or not the circle could be squared. He's not the only one either. There have been a few others.
The earliest efforts to square the circle often centered around the use of inscribed polygons: The idea being that the more sides one adds to the polygon the more area of the circle it would occupy. The hope was that eventually the entire circle would be filled in. For example: An inscribed pentagon would occupy more space within the circle than an inscribed square, and an inscribed hexagon would occupy more space that an inscribed pentagon, and an inscribed octagon would occupy more space within the circle than an inscribed hexagon, and so on. If proven it would also prove that the circle could be squared since polygons can be squared.
To create a square & a circle as close as the solution presented here, one would require the use of inscribed polygons of multiple facets. Archimedes used a 96-gon to set upper & lower bounds for pi of 3.142857 & 3.140845 respectively. Ptolemy used a 360-gon to obtain a value of 3.141666. That’s a super close approximation. But the one generated by these diagrams is even better than that, (3.14164). Huygens defined this number as his upper limit for pi using a square & an octagon. Liu Hui obtained the exact same ratio by inscribing a polygon of 3,072 sides back in the 3rd Century. And Aryabhatta, some time around 500 AD, used a 384-gon to obtain the same close estimate. Imagine trying to draw any one of those with compass & straightedge! I doubt they ever drew any such thing though. Like I said, mathematicians rarely drew these problems out on a sheet of paper in a literal sense.
I will lay out all the diagrams necessary to accomplish this task in detail. You can simply skip these diagrams if you wish & consult the slideshow created for me by Mr. Meisner on the aforementioned website. Here is a link to that slideshow which exemplifies all the steps in quick succession. www.goldennumber.net/wp-content/uploads/2012/05/ricci-squaring-the-circle1.gif

Step #1: Inscribe the Blue Square.
I am not going to bother with the details of this construction. It can be accomplished with compass & straightedge in eleven moves. If you consider the Black Circle & it’s diameter as being already drawn (in Plate #1), then this can be done in only nine moves. This information is common knowledge & can be found on many math related websites. Once this first square has been completed, it’s diagonals can then be extended outwardly to provide the anchoring points for the vertices of all subsequent squares.
I am not going to bother with the details of this construction. It can be accomplished with compass & straightedge in eleven moves. If you consider the Black Circle & it’s diameter as being already drawn (in Plate #1), then this can be done in only nine moves. This information is common knowledge & can be found on many math related websites. Once this first square has been completed, it’s diagonals can then be extended outwardly to provide the anchoring points for the vertices of all subsequent squares.
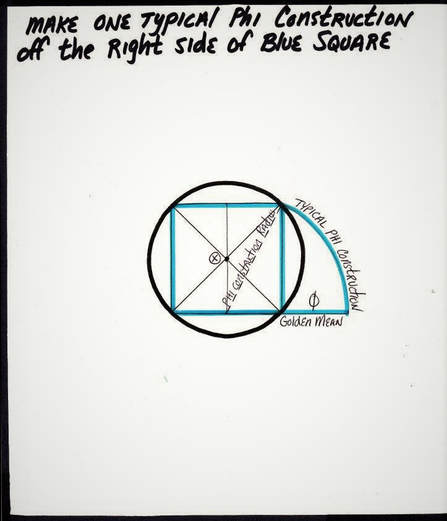
Step #2: Make one standard phi construction off the right side of the Blue Square. Label the endpoint with the letter (Y).
Here, we have the first of three Golden Mean constructions. This is a very special ratio & will be discussed several times throughout the course of this demonstration. It can be found throughout all of creation; from atoms to solar systems to galaxies. Like I said earlier, Gary Meisner is host to an excellent website that delineates many remarkable examples of this ratio's ubiquitous nature. Kepler compared this ratio to a “precious jewel” and said it was one of geometry’s greatest treasures. A friend of Leonardo da Vinci, Luca Pacioli, called it the Divine Proportion. Surely, he was Divinely inspired at the time he settled on that name: For there can be no doubt that it was considered the Divine Proportion long before he was even born.
Here, we have the first of three Golden Mean constructions. This is a very special ratio & will be discussed several times throughout the course of this demonstration. It can be found throughout all of creation; from atoms to solar systems to galaxies. Like I said earlier, Gary Meisner is host to an excellent website that delineates many remarkable examples of this ratio's ubiquitous nature. Kepler compared this ratio to a “precious jewel” and said it was one of geometry’s greatest treasures. A friend of Leonardo da Vinci, Luca Pacioli, called it the Divine Proportion. Surely, he was Divinely inspired at the time he settled on that name: For there can be no doubt that it was considered the Divine Proportion long before he was even born.

Step #3: Using line segment XY as a radius, draw the Pink Circle.
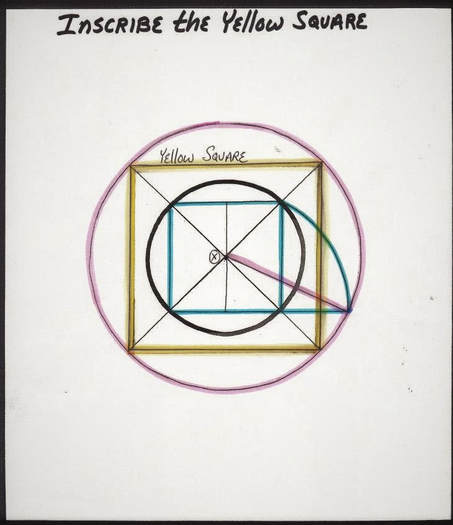
Step #4: Inscribe the Yellow Square.

Step #5: Draw a Green Circle inside the Yellow Square.
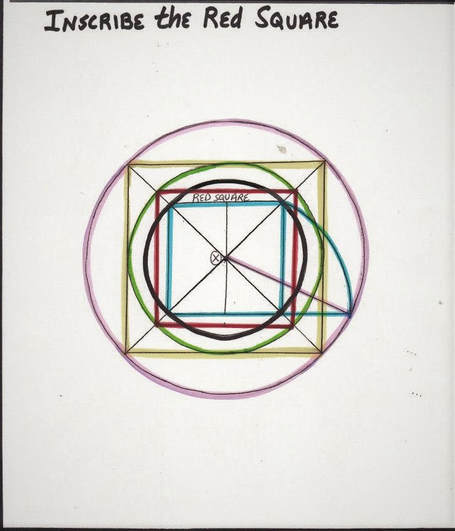
Step #6: Draw the Red Square inside the Green Circle.

Step #7: Make on standard phi construction off the left side of the Red Square. Label the endpoint with the letter (B).
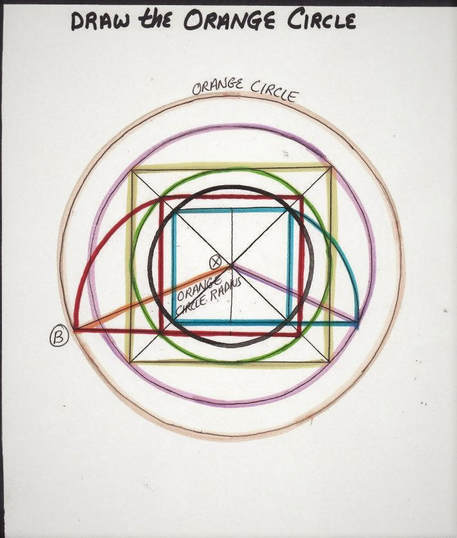
Step #8: Using line segment XB as a radius, draw the Orange Circle.
Now we all have all the shapes required for the presentation.
Now we all have all the shapes required for the presentation.
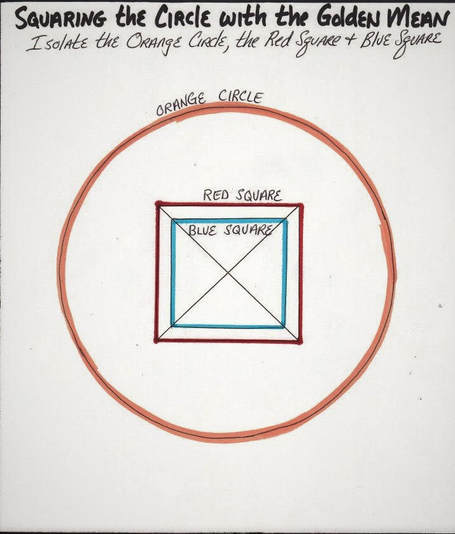
Step #9: Squaring the Circle with Phi:
The next four plates describe an elegant technique for squaring the circle by traditional methods. Here I employ the Divine Proportion, or Golden Mean. First, we need to isolate the following three shapes: The Blue Square, the Red Square, & the Orange Circle.
This simple compass & straightedge method reflects the same ratio generated by the well-known equation, Phi²/5 = Pi/6. The value of the first divided by the value of the second = 1.000015319. When worked in reverse this yields a very tight approximation for pi: [Phi²/5 x 6 = 3.14164]. Obviously, the value of one will never perfectly match the value of the other due to the transcendental nature of Pi (not to mention the irrationality of Phi). There will always be a discrepancy when one interjects Pi into the solution to a quadratic equation & mine is no exception. But it is minor. Algebraically, as opposed to geometrically, one can only hope to come close & this one comes about as close as it gets. I am not saying nobody has come closer, but as yet I don’t know of anyone. If, indeed, there are any, they are certainly very few & far between; especially in utilizing the Golden Mean to accomplish the task. Kepler's Golden Triangle can be used to obtain a value of 3.1446056 for pi, but that's still not as accurate as this.
The next four plates describe an elegant technique for squaring the circle by traditional methods. Here I employ the Divine Proportion, or Golden Mean. First, we need to isolate the following three shapes: The Blue Square, the Red Square, & the Orange Circle.
This simple compass & straightedge method reflects the same ratio generated by the well-known equation, Phi²/5 = Pi/6. The value of the first divided by the value of the second = 1.000015319. When worked in reverse this yields a very tight approximation for pi: [Phi²/5 x 6 = 3.14164]. Obviously, the value of one will never perfectly match the value of the other due to the transcendental nature of Pi (not to mention the irrationality of Phi). There will always be a discrepancy when one interjects Pi into the solution to a quadratic equation & mine is no exception. But it is minor. Algebraically, as opposed to geometrically, one can only hope to come close & this one comes about as close as it gets. I am not saying nobody has come closer, but as yet I don’t know of anyone. If, indeed, there are any, they are certainly very few & far between; especially in utilizing the Golden Mean to accomplish the task. Kepler's Golden Triangle can be used to obtain a value of 3.1446056 for pi, but that's still not as accurate as this.
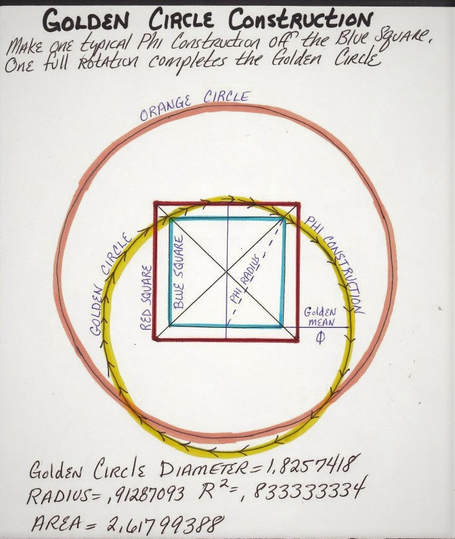
Step #10: Make one standard phi construction from the Blue Square. Continue the arc all the way around in one full rotation to complete the Golden Circle.
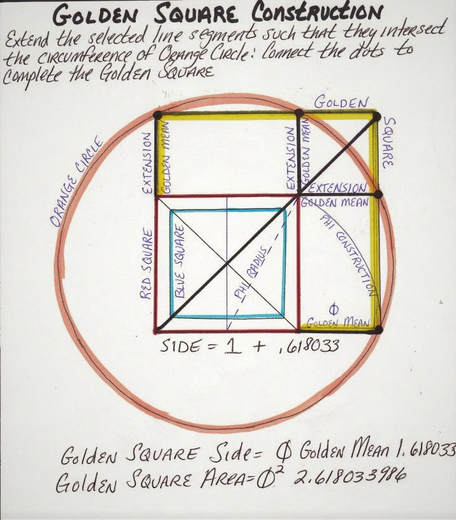
Step #11: Extend the selected lines off the Red Square as shown. Connect the dots to complete the Golden Square.

Step #12: Here we merge the Golden Circle & the Golden Square. This resembles most diagrams that one might typically expect to find when researching this topic.
If we consider the Red Square as a Unit Square [sides = 1; area = 1], the following calculations will result:
Golden Square = Phi (1.618033988). Area = Phi² (2.618033986).
Golden Circle = Radius (0.91287093); Radius² = (.833333334); Area = (2.61799388).
With respect to area there is virtually no difference between these two shapes. Measured in inches the difference is literally microscopic. And even if we convert them into square feet the difference would not be detectable by the naked eye. (About the size of two red blood cells). This construction yields an extremely tight approximation for pi as well: (3.141640784). This is 99.85% accurate for true pi. To illustrate just how significant this is we would need to enlarge the two shapes astronomically. Imagine, for example, you have a planet with a diameter of a thousand miles. According to pi it would take a car racing along at 60 mph more than 52 hours & 21 minutes to circumnavigate the globe at its equator. If we were to extrapolate our travel time using phi instead, the difference between the two times would be less than three seconds!
The fact that we can attain such a high degree of precision without the aid of modern tools & in so few steps sets this construction apart from some of even the most ingenious techniques.
Although these diagrams reveal an extremely tight approximation for pi and this fact most definitely ought to be mentioned, I do not feel it is the most remarkable aspect to be highlighted. It must be remembered that pi was not even used to construct them. For that matter, I didn't use phi either. (By that I mean I didn't use their actual numerical values). The most remarkable thing about these drawings is that they were constructed without the use of any mathematics whatsoever. The instruments employed were primitive & utterly devoid of incremental markings. Actual measurement played no part in it; except, of course, in the proofs.
This procedure is so simple you'd expect to see these drawings surface whenever this subject appeared. Yet, diagrams that demonstrate this phenomenon are practically non-existent. For the life of me I cannot figure out why this is. Now, I have found a few diagrams here & there. But, for the most part, solutions have come in the form of algebraic equations that would be extremely difficult to reproduce in the form of a simple diagram. If we focus solely on constructible illustrations, as opposed to algebraic equations, I'm convinced you won't find a better, or simpler, or more accurate method than the one presented here.
If we consider the Red Square as a Unit Square [sides = 1; area = 1], the following calculations will result:
Golden Square = Phi (1.618033988). Area = Phi² (2.618033986).
Golden Circle = Radius (0.91287093); Radius² = (.833333334); Area = (2.61799388).
With respect to area there is virtually no difference between these two shapes. Measured in inches the difference is literally microscopic. And even if we convert them into square feet the difference would not be detectable by the naked eye. (About the size of two red blood cells). This construction yields an extremely tight approximation for pi as well: (3.141640784). This is 99.85% accurate for true pi. To illustrate just how significant this is we would need to enlarge the two shapes astronomically. Imagine, for example, you have a planet with a diameter of a thousand miles. According to pi it would take a car racing along at 60 mph more than 52 hours & 21 minutes to circumnavigate the globe at its equator. If we were to extrapolate our travel time using phi instead, the difference between the two times would be less than three seconds!
The fact that we can attain such a high degree of precision without the aid of modern tools & in so few steps sets this construction apart from some of even the most ingenious techniques.
Although these diagrams reveal an extremely tight approximation for pi and this fact most definitely ought to be mentioned, I do not feel it is the most remarkable aspect to be highlighted. It must be remembered that pi was not even used to construct them. For that matter, I didn't use phi either. (By that I mean I didn't use their actual numerical values). The most remarkable thing about these drawings is that they were constructed without the use of any mathematics whatsoever. The instruments employed were primitive & utterly devoid of incremental markings. Actual measurement played no part in it; except, of course, in the proofs.
This procedure is so simple you'd expect to see these drawings surface whenever this subject appeared. Yet, diagrams that demonstrate this phenomenon are practically non-existent. For the life of me I cannot figure out why this is. Now, I have found a few diagrams here & there. But, for the most part, solutions have come in the form of algebraic equations that would be extremely difficult to reproduce in the form of a simple diagram. If we focus solely on constructible illustrations, as opposed to algebraic equations, I'm convinced you won't find a better, or simpler, or more accurate method than the one presented here.